Engineering Mechanics Statics 12th Edition Solutions Chapter 5
Successfully reported this slideshow.
Create your free account to read unlimited documents.
Hibbeler chapter5

You are reading a preview.
Create your free account to continue reading.
 1
1
Like this document? Why not share!
- 111 Likes
- Statistics
- Notes
Hibbeler chapter5
- 1. Engineering Mechanics - Statics Chapter 5 Problem 5-1 Draw the free-body diagram of the sphere of weight W resting between the smooth inclined planes. Explain the significance of each force on the diagram. Given: W = 10 lb θ 1 = 105 deg θ 2 = 45 deg Solution: NA, NB force of plane on sphere. W force of gravity on sphere. Problem 5-2 Draw the free-body diagram of the hand punch, which is pinned at A and bears down on the smooth surface at B. Given: F = 8 lb a = 1.5 ft b = 0.2 ft c = 2 ft 340 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 2. Engineering Mechanics - Statics Chapter 5 Solution: Problem 5-3 Draw the free-body diagram of the beam supported at A by a fixed support and at B by a roller. Explain the significance of each force on the diagram. Given: w = 40 lb ft a = 3 ft b = 4 ft θ = 30 deg Solution: A x, A y, MA effect of wall on beam. NB force of roller on beam. wa 2 resultant force of distributed load on beam. Problem 5-4 Draw the free-body diagram of the jib crane AB, which is pin-connected at A and supported by member (link) BC. 341 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 3. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kN = 10 N Given: F = 8 kN a = 3m b = 4m c = 0.4 m d = 3 e = 4 Solution: Problem 5-5 Draw the free-body diagram of the C-bracket supported at A, B, and C by rollers. Explain the significance of each forcce on the diagram. Given: a = 3 ft b = 4 ft θ 1 = 30 deg θ 2 = 20 deg F = 200 lb 342 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 4. Engineering Mechanics - Statics Chapter 5 Solution: NA , NB , NC force of rollers on beam. Problem 5-6 Draw the free-body diagram of the smooth rod of mass M which rests inside the glass. Explain the significance of each force on the diagram. Given: M = 20 gm a = 75 mm b = 200 mm θ = 40 deg Solution: A x , A y , NB force of glass on rod. M(g) N force of gravity on rod. Problem 5-7 Draw the free-body diagram of the "spanner wrench" subjected to the force F. The support at A can be considered a pin, and the surface of contact at B is smooth. Explain the significance of 343 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 5. Engineering Mechanics - Statics Chapter 5 p each force on the diagram. p g Given: F = 20 lb a = 1 in b = 6 in Solution: A x, A y, NB force of cylinder on wrench. Problem 5-8 Draw the free-body diagram of the automobile, which is being towed at constant velocity up the incline using the cable at C. The automobile has a mass M and center of mass at G. The tires are free to roll. Explain the significance of each force on the diagram. Units Used: 3 Mg = 10 kg Given: M = 5 Mg d = 1.50 m a = 0.3 m e = 0.6 m b = 0.75 m θ 1 = 20 deg c = 1m θ 2 = 30 deg g = 9.81 m 2 s 344 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 6. Engineering Mechanics - Statics Chapter 5 Solution: NA, NB force of road on car. F force of cable on car. Mg force of gravity on car. Problem 5-9 Draw the free-body diagram of the uniform bar, which has mass M and center of mass at G. The supports A, B, and C are smooth. Given: M = 100 kg a = 1.75 m b = 1.25 m c = 0.5 m d = 0.2 m g = 9.81 m 2 s Solution: © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 7. Engineering Mechanics - Statics Chapter 5 Problem 5-10 Draw the free-body diagram of the beam, which is pin-connected at A and rocker-supported at B. Given: F = 500 N M = 800 N⋅ m a = 8m b = 4m c = 5m Solution: Problem 5-11 The sphere of weight W rests between the smooth inclined planes. Determine the reaactions at the supports. Given: W = 10 lb θ 1 = 105 deg θ 2 = 45 deg Solution: Initial guesses 346 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 8. Engineering Mechanics - Statics NA = 1 lb Chapter 5 NB = 1 lb Given NB cos ( θ 1 − 90 deg) − NA cos ( θ 2 ) = 0 NA sin ( θ 2 ) − NB sin ( θ 1 − 90 deg) − W = 0 ⎛ NA ⎞ ⎜ ⎟ = Find ( NA , NB) ⎝ NB ⎠ ⎛ NA ⎞ ⎛ 19.3 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ NB ⎠ ⎝ 14.1 ⎠ Problem 5-12 Determine the magnitude of the resultant force acting at pin A of the handpunch. Given: F = 8 lb a = 1.5 ft b = 0.2 ft c = 2 ft Solution: Σ F x = 0; Σ M = 0; Ax − F = 0 Ax = F F a − Ay c = 0 Ay = F Ax = 8 lb a c Ay = 6 lb 347 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 9. Engineering Mechanics - Statics FA = Chapter 5 2 Ax + Ay 2 F A = 10 lb Problem 5-13 The C-bracket is supported at A, B, and C by rollers. Determine the reactions at the supports. Given: a = 3 ft b = 4 ft θ 1 = 30 deg θ 2 = 20 deg F = 200 lb Solution: Initial Guesses: NA = 1 lb NB = 1 lb NC = 1 lb Given NA a − F b = 0 NB sin ( θ 1 ) − NC sin ( θ 2 ) = 0 NB cos ( θ 1 ) + NC cos ( θ 2 ) − NA − F = 0 ⎛ NA ⎞ ⎜ ⎟ ⎜ NB ⎟ = Find ( NA , NB , NC) ⎜N ⎟ ⎝ C⎠ ⎛ NA ⎞ ⎛ 266.7 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ NB ⎟ = ⎜ 208.4 ⎟ lb ⎜ N ⎟ ⎝ 304.6 ⎠ ⎝ C⎠ 348 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 10. Engineering Mechanics - Statics Chapter 5 Problem 5-14 The smooth rod of mass M rests inside the glass. Determine the reactions on the rod. Given: M = 20 gm a = 75 mm b = 200 mm θ = 40 deg g = 9.81 m 2 s Solution: Initial Guesses: Ax = 1 N Ay = 1 N NB = 1 N Given Ax − NB sin ( θ ) = 0 Ay − M g + NB cos ( θ ) = 0 −M g a+b cos ( θ ) + NB b = 0 2 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ = Find ( Ax , Ay , NB) ⎜N ⎟ ⎝ B⎠ ⎛ Ax ⎞ ⎛ 0.066 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 0.117 ⎟ N ⎜ N ⎟ ⎝ 0.103 ⎠ ⎝ B⎠ Problem 5-15 The "spanner wrench" is subjected to the force F. The support at A can be considered a pin, and the surface of contact at B is smooth. Determine the reactions on the spanner wrench. 349 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 11. Engineering Mechanics - Statics Chapter 5 Given: F = 20 lb a = 1 in b = 6 in Solution: Initial Guesses: Ax = 1 lb Ay = 1 lb NB = 1 lb Given − Ax + NB = 0 Ay − F = 0 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ = Find ( Ax , Ay , NB) ⎜N ⎟ ⎝ B⎠ −F ( a + b) + Ax a = 0 ⎛ Ax ⎞ ⎛ 140 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 20 ⎟ lb ⎜ N ⎟ ⎝ 140 ⎠ ⎝ B⎠ Problem 5-16 The automobile is being towed at constant velocity up the incline using the cable at C. The automobile has a mass M and center of mass at G. The tires are free to roll. Determine the reactions on both wheels at A and B and the tension in the cable at C. Units Used: 3 Mg = 10 kg 3 kN = 10 N 350 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 12. Engineering Mechanics - Statics Chapter 5 Given: M = 5 Mg d = 1.50 m a = 0.3 m e = 0.6 m b = 0.75 m θ 1 = 20 deg c = 1m θ 2 = 30 deg g = 9.81 m 2 s Solution: Guesses F = 1 kN NA = 1 kN NB = 1 kN Given NA + NB + F sin ( θ 2 ) − M g cos ( θ 1 ) = 0 −F cos ( θ 2 ) + M g sin ( θ 1 ) = 0 F cos ( θ 2 ) a − F sin ( θ 2 ) b − M g cos ( θ 1 ) c − M g sin ( θ 1 ) e + NB( c + d) = 0 ⎛F ⎞ ⎜ ⎟ ⎜ NA ⎟ = Find ( F , NA , NB) ⎜ NB ⎟ ⎝ ⎠ ⎛ F ⎞ ⎛ 19.37 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ NA ⎟ = ⎜ 13.05 ⎟ kN ⎜ NB ⎟ ⎝ 23.36 ⎠ ⎝ ⎠ Problem 5-17 The uniform bar has mass M and center of mass at G. The supports A, B, and C are smooth. Determine the reactions at the points of contact at A, B, and C. Given: M = 100 kg a = 1.75 m b = 1.25 m 351 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 13. Engineering Mechanics - Statics Chapter 5 c = 0.5 m d = 0.2 m θ = 30 deg g = 9.81 m 2 s Solution: The initial guesses: NA = 20 N NB = 30 N NC = 40 N Given ΣMA = 0; + ↑Σ Fy = 0; + ↑Σ Fy = 0; −M g cos ( θ ) a − M g sin ( θ ) d + NB sin ( θ ) d + NC( a + b) = 0 2 NB − M g + NC cos ( θ ) = 0 NA − NC sin ( θ ) = 0 ⎛ NC ⎞ ⎜ ⎟ ⎜ NB ⎟ = Find ( NC , NB , NA) ⎜N ⎟ ⎝ A⎠ ⎛ NC ⎞ ⎛ 493 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ NB ⎟ = ⎜ 554 ⎟ N ⎜ N ⎟ ⎝ 247 ⎠ ⎝ A⎠ Problem 5-18 The beam is pin-connected at A and rocker-supported at B. Determine the reactions at the pin A and at the roller at B. Given: F = 500 N M = 800 N⋅ m a = 8m 352 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 14. Engineering Mechanics - Statics Chapter 5 b = 4m c = 5m Solution: ⎞ ⎟ ⎝ a + b⎠ α = atan ⎛ ⎜ c ΣMA = 0; −F a cos ( α ) By = − M + By a = 0 F a + M cos ( α ) cos ( α ) a B y = 642 N + → Σ Fx = 0; + ↑Σ Fy = 0; − Ax + F sin ( α ) = 0 Ax = F sin ( α ) Ax = 192 N − Ay − F cos ( α ) + B y = 0 Ay = −F cos ( α ) + By Ay = 180 N Problem 5-19 Determine the magnitude of the reactions on the beam at A and B. Neglect the thickness of the beam. Given: F 1 = 600 N F 2 = 400 N θ = 15 deg a = 4m b = 8m c = 3 d = 4 Solution: ΣMA = 0; B y( a + b) − F2 cos ( θ ) ( a + b) − F1 a = 0 353 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 15. Engineering Mechanics - Statics By = + → Σ Fx = 0; Chapter 5 F 2 cos ( θ ) ( a + b) + F 1 a a+b Ax − F 2 sin ( θ ) = 0 Ax = F2 sin ( θ ) + ↑Σ Fy = 0; B y = 586 N Ax = 104 N Ay − F 2 cos ( θ ) + B y − F1 = 0 Ay = F2 cos ( θ ) − B y + F1 FA = 2 Ax + Ay 2 Ay = 400 N F A = 413 N Problem 5-20 Determine the reactions at the supports. Given: w = 250 lb ft a = 6 ft b = 6 ft c = 6 ft Solution: Guesses Ax = 1 lb Ay = 1 lb B y = 1 lb Given Ax = 0 wa Ay + B y − w( a + b) − ⎛ a ⎞ − w b⎛ b ⎞ − 1 w c⎛b + ⎜ ⎟ ⎜ ⎟ ⎜ ⎝ 2⎠ ⎝ 2⎠ 2 ⎝ 1 2 wc = 0 c⎞ ⎟ + By b = 0 3⎠ 354 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 16. Engineering Mechanics - Statics Chapter 5 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ = Find ( Ax , Ay , By) ⎜B ⎟ ⎝ y⎠ ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 2750 ⎟ lb ⎜ B ⎟ ⎝ 1000 ⎠ ⎝ y⎠ Problem 5-21 When holding the stone of weight W in equilibrium, the humerus H, assumed to be smooth, exerts normal forces FC and F A on the radius C and ulna A as shown. Determine these forces and the force F B that the biceps B exerts on the radius for equilibrium. The stone has a center of mass at G. Neglect the weight of the arm. Given: W = 5 lb θ = 75 deg a = 2 in b = 0.8 in c = 14 in Solution: ΣMB = 0; − W ( c − a) + F A a = 0 FA = W ⎛ c − a⎞ ⎜ ⎟ ⎝ a ⎠ F A = 30 lb + ↑Σ Fy = 0; F B sin ( θ ) − W − FA = 0 FB = W + FA sin ( θ ) F B = 36.2 lb + → Σ Fx = 0; F C − F B cos ( θ ) = 0 355 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 17. Engineering Mechanics - Statics Chapter 5 F C = FB cos ( θ ) F C = 9.378 lb Problem 5-22 The uniform door has a weight W and a center of gravity at G. Determine the reactions at the hinges if the hinge at A supports only a horizontal reaction on the door, whereas the hinge at B exerts both horizontal and vertical reactions. Given: W = 100 lb a = 3 ft b = 3 ft c = 0.5 ft d = 2 ft Solution: ΣMB = 0; W d − A x ( a + b) = 0 Ax = W ⎛ d ⎞ ⎜ ⎟ ⎝ a + b⎠ Ax = 33.3 lb ΣF x = 0; Bx = Ax B x = 33.3 lb ΣF y = 0; By = W B y = 100 lb Problem 5-23 The ramp of a ship has weight W and center of gravity at G. Determine the cable force in CD needed to just start lifting the ramp, (i.e., so the reaction at B becomes zero). Also, determine the horizontal and vertical components of force at the hinge (pin) at A. 356 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 18. Engineering Mechanics - Statics Chapter 5 Given: W = 200 lb a = 4 ft θ = 30 deg b = 3 ft φ = 20 deg c = 6 ft Solution: ΣMA = 0; −F CD cos ( θ ) ( b + c) cos ( φ ) + FCD sin ( θ ) ( b + c) sin ( φ ) + W c cos ( φ ) = 0 F CD = W c cos ( φ ) ( b + c) ( cos ( θ ) cos ( φ ) − sin ( θ ) sin ( φ ) ) + → Σ Fx = 0; F CD sin ( θ ) − A x = 0 Ax = FCD sin ( θ ) + ↑Σ Fy = 0; F CD = 195 lb Ax = 97.5 lb Ay − W + F CD cos ( θ ) = 0 Ay = W − FCD cos ( θ ) Ay = 31.2 lb Problem 5-24 The drainpipe of mass M is held in the tines of the fork lift. Determine the normal forces at A and B as functions of the blade angle θ and plot the results of force (ordinate) versus θ (abscissa) for 0 ≤ θ ≤ 90 deg. 357 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 19. Engineering Mechanics - Statics Chapter 5 Units used: 3 Mg = 10 kg Given: M = 1.4 Mg a = 0.4 m g = 9.81 m 2 s Solution: θ = 0 .. 90 NA ( θ ) = M g sin ( θ deg) 3 10 NB ( θ ) = M g cos ( θ deg) 3 10 Force in kN 15 NA( θ )10 NB( θ ) 5 0 0 20 40 60 80 100 θ Angle in Degrees Problem 5-25 While slowly walking, a man having a total mass M places all his weight on one foot. Assuming that the normal force NC of the ground acts on his foot at C, determine the resultant vertical compressive force F B which the tibia T exerts on the astragalus B, and the vertical tension FA in the achilles tendon A at the instant shown. 358 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 20. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kN = 10 N Given: M = 80 kg a = 15 mm b = 5 mm c = 20 mm d = 100 mm Solution: NC = M g NC = 785 N ΣMA = 0; −F B c + NC ( c + d) = 0 F B = NC ⎛ c + d⎞ ⎜ ⎟ ⎝ c ⎠ F B = 4.71 kN ΣF y = 0; F A − FB + NC = 0 F A = F B − NC F A = 3.92 kN Problem 5-26 Determine the reactions at the roller A and pin B. 359 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 21. Engineering Mechanics - Statics Chapter 5 Given: M = 800 lb ft c = 3 ft F = 390 lb d = 5 a = 8 ft e = 12 b = 4 ft θ = 30 deg Solution: Guesses R A = 1 lb B x = 1 lb B y = 1 lb Given R A sin ( θ ) + B x − ⎛ d ⎞F = 0 ⎜ 2 2⎟ ⎝ e +d ⎠ R A cos ( θ ) + By − e ⎛ ⎞ ⎜ 2 2 ⎟F = 0 ⎝ e +d ⎠ M − R A cos ( θ ) ( a + b) + Bx c = 0 ⎛ RA ⎞ ⎜ ⎟ ⎜ Bx ⎟ = Find ( RA , Bx , By) ⎜B ⎟ ⎝ y⎠ R A = 105.1 lb ⎛ Bx ⎞ ⎛ 97.4 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ By ⎠ ⎝ 269 ⎠ Problem 5-27 The platform assembly has weight W1 and center of gravity at G1. If it is intended to support a maximum load W2 placed at point G2,,determine the smallest counterweight W that should be placed at B in order to prevent the platform from tipping over. Given: W1 = 250 lb a = 1 ft c = 1 ft e = 6 ft W2 = 400 lb b = 6 ft d = 8 ft f = 2 ft 360 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 22. Engineering Mechanics - Statics Chapter 5 Solution: When tipping occurs, R c = 0 ΣMD = 0; −W2 f + W1 c + WB ( b + c) = 0 WB = W2 f − W1 c b+c WB = 78.6 lb Problem 5-28 The articulated crane boom has a weight W and mass center at G. If it supports a load L, determine the force acting at the pin A and the compression in the hydraulic cylinder BC when the boom is in the position shown. Units Used: 3 kip = 10 lb Given: W = 125 lb 361 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
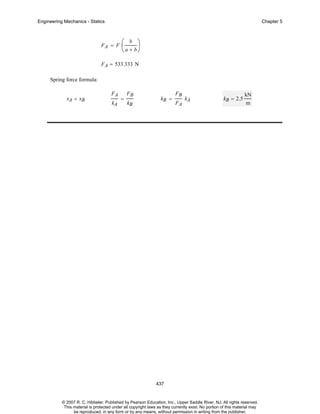
- 23. Engineering Mechanics - Statics Chapter 5 L = 600 lb a = 4 ft b = 1 ft c = 1 ft d = 8 ft θ = 40 deg Solution: Guesses Given Ax = 1 lb Ay = 1 lb F B = 1 lb − Ax + F B cos ( θ ) = 0 − Ay + F B sin ( θ ) − W − L = 0 F B cos ( θ ) b + F B sin ( θ ) c − W a − L( d + c) = 0 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ = Find ( Ax , Ay , FB) ⎜F ⎟ ⎝ B⎠ F B = 4.19 kip ⎛ Ax ⎞ ⎛ 3.208 ⎞ ⎜ ⎟=⎜ ⎟ kip ⎝ Ay ⎠ ⎝ 1.967 ⎠ Problem 5-29 The device is used to hold an elevator door open. If the spring has stiffness k and it is compressed a distnace δ, determine the horizontal and vertical components of reaction at the pin A and the resultant force at the wheel bearing B. Given: N m b = 125 mm δ = 0.2 m c = 100 mm a = 150 mm θ = 30 deg k = 40 362 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 24. Engineering Mechanics - Statics Solution: ΣMA = 0; Chapter 5 F s = kδ −F s a + F B cos ( θ ) ( a + b) − F B sin ( θ ) c = 0 FB = Fs a cos ( θ ) ( a + b) − sin ( θ ) c F B = 6.378 N + → Σ Fx = 0; Ax − F B sin ( θ ) = 0 Ax = FB sin ( θ ) Ax = 3.189 N + ↑Σ Fy = 0; Ay − F s + F B cos ( θ ) = 0 Ay = Fs − FB cos ( θ ) Ay = 2.477 N Problem 5-30 Determine the reactions on the bent rod which is supported by a smooth surface at B and by a collar at A, which is fixed to the rod and is free to slide over the fixed inclined rod. Given: F = 100 lb M = 200 lb ft a = 3 ft b = 3 ft c = 2ft 363 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 25. Engineering Mechanics - Statics Chapter 5 d = 3 e = 4 f = 12 g = 5 Solution: Initial Guesses: NA = 20 lb NB = 10 lb MA = 30 lb ft Given f g ⎞ ⎞ ⎛ ⎛ ⎜ 2 2 ⎟ ( a + b) − NB ⎜ 2 2 ⎟ c = 0 ⎝ f +g ⎠ ⎝ f +g ⎠ ΣMA = 0; MA − F a − M + NB ΣF x = 0; NA e g ⎞ ⎞ ⎛ ⎛ ⎜ 2 2 ⎟ − NB ⎜ 2 2 ⎟ = 0 ⎝ e +d ⎠ ⎝ f +g ⎠ ΣF y = 0; NA f ⎞ ⎛ d ⎞+N ⎛ B⎜ ⎜ 2 2⎟ ⎟−F=0 2 2 ⎝ e +d ⎠ ⎝ f +g ⎠ ⎛ NA ⎞ ⎜ ⎟ ⎜ NB ⎟ = Find ( NA , NB , MA) ⎜M ⎟ ⎝ A⎠ ⎛ NA ⎞ ⎛ 39.7 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ NB ⎠ ⎝ 82.5 ⎠ MA = 106 lb⋅ ft Problem 5-31 The cantilevered jib crane is used to support the load F. If the trolley T can be placed anywhere in the range x1 ≤ x ≤ x2, determine the maximum magnitude of reaction at the supports A and B. Note that the supports are collars that allow the crane to rotate freely about the vertical axis. The collar at B supports a force in the vertical direction, whereas the one at A does not. Units Used: kip = 1000 lb Given: F = 780 lb 364 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 26. Engineering Mechanics - Statics Chapter 5 a = 4 ft b = 8 ft x1 = 1.5 ft x2 = 7.5 ft Solution: The maximum occurs when x = x2 ΣMA = 0; −F x2 + B x a = 0 Bx = F x2 a 3 B x = 1.462 × 10 lb + → Σ Fx = 0; + ↑Σ Fy = 0; FB = Ax − Bx = 0 By − F = 0 2 Bx + By Ax = Bx By = F 2 3 Ax = 1.462 × 10 lb B y = 780 lb F B = 1.657 kip Problem 5-32 The uniform rod AB has weight W. Determine the force in the cable when the rod is in the position shown. Given: W = 15 lb L = 5 ft 365 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 27. Engineering Mechanics - Statics Chapter 5 θ 1 = 30 deg θ 2 = 10 deg Solution: ΣMA = 0; NB L sin ( θ 1 + θ 2 ) − W NB = ⎛ L ⎞ cos ( θ + θ ) = 0 ⎜ ⎟ 1 2 ⎝2⎠ W cos ( θ 1 + θ 2 ) 2 sin ( θ 1 + θ 2 ) NB = 8.938 lb ΣF x = 0; T cos ( θ 2 ) − NB T = NB cos ( θ 2 ) T = 9.08 lb Problem 5-33 The power pole supports the three lines, each line exerting a vertical force on the pole due to its weight as shown. Determine the reactions at the fixed support D. If it is possible for wind or ice to snap the lines, determine which line(s) when removed create(s) a condition for the greatest moment reaction at D. Units Used: 3 kip = 10 lb 366 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 28. Engineering Mechanics - Statics Chapter 5 Given: W1 = 800 lb W2 = 450 lb W3 = 400 lb a = 2ft b = 4 ft c = 3 ft Solution: + → Σ Fx = 0; + ↑Σ Fy = 0; Dx = 0 Dy − ( W1 + W2 + W3 ) = 0 Dy = W1 + W2 + W3 ΣMD = 0; Dy = 1.65 kip −W2 b − W3 c + W1 a + MD = 0 MD = W2 b + W3 c − W1 a MD = 1.4 kip⋅ ft Examine all cases. For these numbers we require line 1 to snap. MDmax = W2 b + W3 c MDmax = 3 kip⋅ ft Problem 5-34 The picnic table has a weight WT and a center of gravity at GT . If a man weighing WM has a center of gravity at GM and sits down in the centered position shown, determine the vertical reaction at each of the two legs at B.Neglect the thickness of the legs. What can you conclude from the results? Given: WT = 50 lb 367 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 29. Engineering Mechanics - Statics Chapter 5 WM = 225 lb a = 6 in b = 20 in c = 20 in Solution: ΣMA = 0; 2 NB( b + c) + WM a − WT b = 0 NB = WT b − WM a 2 ( b + c) NB = −4.37 lb Since NB has a negative sign, the table will tip over. Problem 5-35 If the wheelbarrow and its contents have a mass of M and center of mass at G, determine the magnitude of the resultant force which the man must exert on each of the two handles in order to hold the wheelbarrow in equilibrium. Given: M = 60 kg a = 0.6 m b = 0.5 m c = 0.9 m d = 0.5 m g = 9.81 m 2 s 368 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 30. Engineering Mechanics - Statics Chapter 5 Solution: ΣMB = 0; − Ay ( b + c) + M g c = 0 Ay = Mgc b+c Ay = 378.386 N + → Σ Fx = 0; B x = 0N + Ay − M g + 2 By = 0 ↑Σ Fy = 0; By = Bx = 0 M g − Ay 2 B y = 105.107 N Problem 5-36 The man has weight W and stands at the center of the plank. If the planes at A and B are smooth, determine the tension in the cord in terms of W and θ. Solution: ΣMB = 0; W L cos ( φ ) − NA L cos ( φ ) = 0 2 NA = ΣF x = 0; W 2 T cos ( θ ) − NB sin ( θ ) = 0 (1) 369 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 31. Engineering Mechanics - Statics Chapter 5 T sin ( θ ) + NB cos ( θ ) + NA − W = 0 ΣF y = 0; (2) Solving Eqs. (1) and (2) yields: T= W sin ( θ ) 2 NB = W cos ( θ ) 2 Problem 5-37 When no force is applied to the brake pedal of the lightweight truck, the retainer spring AB keeps the pedal in contact with the smooth brake light switch at C. If the force on the switch is F , determine the unstretched length of the spring if the stiffness of the spring is k. Given: F = 3N k = 80 N m a = 100 mm b = 50 mm c = 40 mm d = 10 mm θ = 30 deg Solution: ΣMD = 0; F s b − F cos ( θ ) c − F sin ( θ ) d = 0 Fs = F cos ( θ ) c + sin ( θ ) d b Fs = k x x = L0 = a − x Fs k F s = 2.378 N x = 29.73 mm L0 = 70.3 mm 370 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 32. Engineering Mechanics - Statics Chapter 5 Problem 5-38 The telephone pole of negligible thickness is subjected to the force F directed as shown. It is supported by the cable BCD and can be assumed pinned at its base A. In order to provide clearance for a sidewalk right of way, where D is located, the strut CE is attached at C, as shown by the dashed lines (cable segment CD is removed). If the tension in CD' is to be twice the tension in BCD, determine the height h for placement of the strut CE. Given: F = 80 lb θ = 30 deg a = 30 ft b = 10 ft Solution: + ΣMA = 0; −F cos ( θ ) a + b ⎛ ⎞ ⎜ 2 2 ⎟ TBCD a = 0 ⎝ a +b ⎠ TBCD = F cos ( θ ) 2 2 a +b b TBCD = 219.089 lb Require TCD' = 2 TBCD + ΣMA = 0; TCD' = 438.178 lb TCD' d − F cos ( θ ) a = 0 d = Fa ⎛ cos ( θ ) ⎞ ⎜T ⎟ ⎝ CD' ⎠ d = 4.7434 ft Geometry: a−h a = d b h = a−a ⎛ d⎞ ⎜ ⎟ ⎝ b⎠ h = 15.8 ft 371 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 33. Engineering Mechanics - Statics Chapter 5 Problem 5-39 The worker uses the hand truck to move material down the ramp. If the truck and its contents are held in the position shown and have weight W with center of gravity at G, determine the resultant normal force of both wheels on the ground A and the magnitude of the force required at the grip B. Given: W = 100 lb e = 1.5 ft a = 1 ft f = 0.5 ft b = 1.5 ft θ = 60 deg c = 2 ft φ = 30 deg d = 1.75 ft Solution: ΣMB = 0; NA cos ( θ − φ ) ( b + c + d) + NA sin ( θ − φ ) ( a − f) − W cos ( θ ) ( b + c) − W sin ( θ ) ( e + a) = 0 NA = W cos ( θ ) ( b + c) + W sin ( θ ) ( e + a) cos ( θ − φ ) ( b + c + d) + sin ( θ − φ ) ( a − f) NA = 81.621 lb ΣF x = 0; −B x + NA sin ( φ ) = 0 B x = NA sin ( φ ) B x = 40.811 lb ΣF y = 0; B y + NA ( cos ( φ ) − W = 0) B y = W − NA cos ( φ ) B y = 29.314 lb FB = 2 Bx + By 2 F B = 50.2 lb 372 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 34. Engineering Mechanics - Statics Chapter 5 T sin ( θ ) + NB cos ( θ ) + NA − W = 0 ΣF y = 0; (2) Solving Eqs. (1) and (2) yields: T= W sin ( θ ) 2 NB = W cos ( θ ) 2 Problem 5-37 When no force is applied to the brake pedal of the lightweight truck, the retainer spring AB keeps the pedal in contact with the smooth brake light switch at C. If the force on the switch is F , determine the unstretched length of the spring if the stiffness of the spring is k. Given: F = 3N k = 80 N m a = 100 mm b = 50 mm c = 40 mm d = 10 mm θ = 30 deg Solution: ΣMD = 0; F s b − F cos ( θ ) c − F sin ( θ ) d = 0 Fs = F cos ( θ ) c + sin ( θ ) d b Fs = k x x = L0 = a − x Fs k F s = 2.378 N x = 29.73 mm L0 = 70.3 mm 373 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 35. Engineering Mechanics - Statics Chapter 5 The shelf supports the electric motor which has mass m1 and mass center at Gm. The platform upon which it rests has mass m2 and mass center at Gp. Assuming that a single bolt B holds the shelf up and the bracket bears against the smooth wall at A, determine this normal force at A and the horizontal and vertical components of reaction of the bolt B on the bracket. Given: m1 = 15 kg c = 50 mm m2 = 4 kg d = 200 mm a = 60 mm e = 150 mm b = 40 mm g = 9.81 m 2 s Solution: ΣMA = 0; B x a − m2 g d − m1 g( d + e) = 0 Bx = g + → Σ Fx = 0; m2 d + m1 ( d + e) B x = 989 N a Ax − Bx = 0 Ax = Bx + ↑Σ Fy = 0; Ax = 989 N B y − m2 g − m1 g = 0 B y = m2 g + m1 g B y = 186 N Problem 5-42 A cantilever beam, having an extended length L, is subjected to a vertical force F. Assuming that the wall resists this load with linearly varying distributed loads over the length a of the beam portion inside the wall, determine the intensities w1 and w2 for equilibrium. 374 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 36. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kN = 10 N Given: F = 500 N a = 0.15 m L = 3m Solution: The initial guesses w1 = 1 kN m w2 = 1 kN m Given + ↑Σ Fy = 0; ΣMA = 0; ⎛ w1 ⎞ ⎜ ⎟ = Find ( w1 , w2) ⎝ w2 ⎠ 1 1 w1 a − w2 a − F = 0 2 2 −F L − 1 2 1 ⎛ 2 a⎞ ⎟ + w2 a⎜ ⎟ = 0 ⎝ 3⎠ 2 ⎝ 3 ⎠ w1 a⎛ ⎜ a⎞ ⎛ w1 ⎞ ⎛ 413 ⎞ kN ⎜ ⎟=⎜ ⎟ ⎝ w2 ⎠ ⎝ 407 ⎠ m Problem 5-43 The upper portion of the crane boom consists of the jib AB, which is supported by the pin at A, the guy line BC, and the backstay CD, each cable being separately attached to the mast at C. If the load F is supported by the hoist line, which passes over the pulley at B, determine the magnitude of the resultant force the pin exerts on the jib at A for equilibrium, the tension in the guy line BC, and the tension T in the hoist line. Neglect the weight of the jib. The pulley at B has a radius of r. 375 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 37. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kN = 10 N Given: F = 5 kN r = 0.1 m a = r b = 1.5 m c = 5m Solution: From pulley, tension in the hoist line is ΣMB = 0; T( a) − F( r) = 0 T = F r T = 5 kN a From the jib, ΣMA = 0; b+a −F ( c) + TBC c=0 2 c + ( b + a) 2 c + ( b + a) TBC = F + ↑Σ Fy = 0; 2 2 TBC = 16.406 kN b+a b+a ⎤−F=0 ⎢ 2 2⎥ ⎣ c + ( b + a) ⎦ − Ay + TBC ⎡ b+a ⎤−F ⎢ 2 2⎥ ⎣ c + ( b + a) ⎦ Ay = TBC⎡ + → Σ Fx = 0; Ay = 0 kN ⎤−F=0 ⎣ c + ( b + a) ⎦ Ax − TBC ⎡ ⎢ c 2 2⎥ 376 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 38. Engineering Mechanics - Statics Chapter 5 c Ax = TBC 2 c + ( b + a) FA = 2 Ax + Ay +F 2 2 Ax = 20.6 kN F A = 20.6 kN Problem 5-44 The mobile crane has weight W1 and center of gravity at G1; the boom has weight W2 and center of gravity at G2. Determine the smallest angle of tilt θ of the boom, without causing the crane to overturn if the suspended load has weight W. Neglect the thickness of the tracks at A and B. Given: W1 = 120000 lb W2 = 30000 lb W = 40000 lb a = 4 ft b = 6 ft c = 3 ft d = 12 ft e = 15 ft Solution: When tipping occurs, R A = 0 ΣMB = 0; −W2 ( d cos ( θ ) − c) − W⎡( d + e)cos ( θ ) − c⎤ + W1 ( b + c) = 0 ⎣ ⎦ ⎡W2 c + W c + W1 ( b + c) ⎤ ⎥ ⎣ W2 d + W ( d + e) ⎦ θ = acos ⎢ θ = 26.4 deg 377 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 39. Engineering Mechanics - Statics Chapter 5 Problem 5-45 The mobile crane has weight W1 and center of gravity at G1; the boom has weight W2 and center of gravity at G2. If the suspended load has weight W determine the normal reactions at the tracks A and B. For the calculation, neglect the thickness of the tracks . Units Used: 3 kip = 10 lb Given: W1 = 120000 lb a = 4 ft W2 = 30000 lb b = 6 ft W = 16000 lb c = 3 ft θ = 30 deg d = 12 ft e = 15 ft Solution: ΣMB = 0; −W2 ( d cos ( θ ) − c) − W⎡( d + e)cos ( θ ) − c⎤ − RA( a + b + c) + W1 ( b + c) = 0 ⎣ ⎦ RA = + ↑ −W2 ( d cos ( θ ) − c) − W⎡( d + e)cos ( θ ) − c⎤ + W1 ( b + c) ⎣ ⎦ Σ F y = 0; a+b+c R A = 40.9 kip R A + RB − W1 − W2 − W = 0 R B = −R A + W1 + W2 + W R B = 125 kip Problem 5-46 The man attempts to support the load of boards having a weight W and a center of gravity at G. If he is standing on a smooth floor, determine the smallest angle θ at which he can hold them up in the position shown. Neglect his weight . 378 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 40. Engineering Mechanics - Statics Chapter 5 Given: a = 0.5 ft b = 3 ft c = 4 ft d = 4 ft Solution: ΣMB = 0; −NA( a + b) + W( b − c cos ( θ ) ) = 0 As θ becomes smaller, NA goes to 0 so that, cos ( θ ) = b c b⎞ θ = acos ⎛ ⎟ ⎜ ⎝c⎠ θ = 41.4 deg Problem 5-47 The motor has a weight W. Determine the force that each of the chains exerts on the supporting hooks at A, B, and C. Neglect the size of the hooks and the thickness of the beam. 379 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 41. Engineering Mechanics - Statics Chapter 5 Given: W = 850 lb a = 0.5 ft b = 1 ft c = 1.5 ft θ 1 = 10 deg θ 2 = 30 deg θ 3 = 10 deg Solution: Guesses F A = 1 lb F B = 1 lb F C = 1 lb Given ΣMB = 0; F A cos ( θ 3 ) b + W a − FC cos ( θ 1 ) ( a + c) = 0 ΣF x = 0; F C sin ( θ 1 ) − F B sin ( θ 2 ) − FA sin ( θ 3 ) = 0 ΣF y = 0; W − F A cos ( θ 3 ) − F B cos ( θ 2 ) − F C cos ( θ 1 ) = 0 ⎛ FA ⎞ ⎜ ⎟ ⎜ FB ⎟ = Find ( FA , FB , FC) ⎜F ⎟ ⎝ C⎠ ⎛ FA ⎞ ⎛ 432 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ FB ⎟ = ⎜ −0 ⎟ lb ⎜ F ⎟ ⎝ 432 ⎠ ⎝ C⎠ 380 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 42. Engineering Mechanics - Statics Chapter 5 Problem 5-48 The boom supports the two vertical loads. Neglect the size of the collars at D and B and the thickness of the boom, and compute the horizontal and vertical components of force at the pin A and the force in cable CB. Given: F 1 = 800 N F 2 = 350 N a = 1.5 m b = 1m c = 3 d = 4 θ = 30 deg Solution: ΣMA = 0; −F 1 a cos ( θ ) − F2 ( a + b) cos ( θ ) + d 2 2 FCB ( a + b) sin ( θ ) + c +d F CB d 2 c +d Ax = 2 FCB ( a + b) cos ( θ ) = 0 F CB = 0 d 2 2 F CB = 782 N d sin ( θ ) ( a + b) + c cos ( θ ) ( a + b) Ax − 2 c +d 2 2 ⎡F1 a + F2 ( a + b)⎤ cos ( θ ) c + d ⎣ ⎦ = + → Σ Fx = 0; c 2 Ax = 625 N F CB c +d + ↑ Σ F y = 0; Ay − F1 − F2 + c 2 c +d Ay = F1 + F2 − 2 F CB = 0 c 2 2 FCB Ay = 681 N c +d Problem 5-49 The boom is intended to support two vertical loads F1 and F 2. If the cable CB can sustain a 381 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 43. Engineering Mechanics - Statics Chapter 5 maximum load Tmax before it fails, determine the critical loads if F1 = 2F2. Also, what is the magnitude of the maximum reaction at pin A? Units Used: 3 kN = 10 N Given: Tmax = 1500 N a = 1.5 m b = 1m c = 3 d = 4 θ = 30deg Solution: ΣMA = 0; F1 = 2 F2 −2 F 2 a cos ( θ ) − F2 ( a + b) cos ( θ ) + d 2 2 Tmax( a + b) sin ( θ ) + c +d F2 = ( a + b)Tmax( d sin ( θ ) + c cos ( θ ) ) 2 F1 = 2 F2 + → ΣFx = 0; Ax − d 2 c +d Ax = 2 2 Tmax( a + b) cos ( θ ) = 0 F 1 = 1.448 kN Tmax = 0 d 2 2 c +d F 2 = 724 N c + d cos ( θ ) ( 3 a + b) 2 c 2 Ax = 1.20 kN Tmax c +d + ↑Σ Fy = 0; Ay − F2 − F1 + c 2 c +d 2 Tmax = 0 382 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 44. Engineering Mechanics - Statics Chapter 5 c Ay = F2 + F1 − 2 2 Ay = 1.27 kN Tmax c +d 2 FA = Ax + Ay 2 F A = 1.749 kN Problem 5-50 The uniform rod of length L and weight W is supported on the smooth planes. Determine its position θ for equilibrium. Neglect the thickness of the rod. Solution: L cos ( θ ) + NA cos ( φ − θ ) L = 0 ΣMB = 0; −W ΣMA = 0; W ΣF x = 0; W cos ( θ ) NB sin ( ψ) − NA sin ( φ ) = 0 W cos ( θ ) 2 cos ( ψ + θ ) L 2 2 NA = cos ( θ ) − NB cos ( ψ + θ ) L = 0 sin ( ψ) − W cos ( θ ) 2 cos ( φ − θ ) NB = 2 cos ( φ − θ ) W cos ( θ ) 2 cos ( ψ + θ ) sin ( φ ) = 0 sin ( ψ) cos ( φ − θ ) − sin ( φ ) cos ( ψ + θ ) = 0 sin ( ψ) ( cos ( φ ) cos ( θ ) + sin ( φ ) sin ( θ ) ) − sin ( φ ) ( cos ( ψ) cos ( θ ) − sin ( ψ) sin ( θ ) ) = 0 2 sin ( ψ) sin ( φ ) sin ( θ ) = ( sin ( φ ) cos ( ψ) − sin ( ψ) cos ( φ ) ) cos ( θ ) tan ( θ ) = sin ( φ ) cos ( ψ) − sin ( ψ) cos ( φ ) 2 sin ( ψ) sin ( φ ) = cot ( ψ) − cot ( φ ) 2 ⎛ cot ( ψ) − cot ( φ ) ⎞ ⎟ 2 ⎝ ⎠ θ = atan ⎜ 383 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 45. Engineering Mechanics - Statics Chapter 5 Problem 5-51 The toggle switch consists of a cocking lever that is pinned to a fixed frame at A and held in place by the spring which has unstretched length δ. Determine the magnitude of the resultant force at A and the normal force on the peg at B when the lever is in the position shown. Given: δ = 200 mm k = 5 N m a = 100 mm b = 300 mm c = 300 mm θ = 30 deg Solution: Using the law of cosines and the law of sines c + ( a + b) − 2 c( a + b) cos ( 180 deg − θ ) 2 l = sin ( φ ) c = 2 sin ( 180 deg − θ ) l Fs = k s = k (l − δ ) ΣMA = 0; −F s sin ( φ ) ( a + b) + NB a = 0 ⎛ sin (180 deg − θ ) ⎞ ⎟ l ⎝ ⎠ φ = asin ⎜c φ = 12.808 deg F s = k( l − δ ) F s = 2.3832 N NB = F s sin ( φ ) a+b a NB = 2.11 N ΣF x = 0; Ax − F s cos ( φ ) = 0 Ax = Fs cos ( φ ) Ax = 2.3239 N ΣF y = 0; Ay + NB − Fs sin ( φ ) = 0 Ay = Fs sin ( φ ) − NB Ay = −1.5850 N FA = 2 Ax + Ay 2 F A = 2.813 N 384 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 46. Engineering Mechanics - Statics Chapter 5 Problem 5-52 The rigid beam of negligible weight is supported horizontally by two springs and a pin. If the springs are uncompressed when the load is removed, determine the force in each spring when the load P is applied. Also, compute the vertical deflection of end C. Assume the spring stiffness k is large enough so that only small deflections occur. Hint: The beam rotates about A so the deflections in the springs can be related. Solution: ΣMA = 0; 3 F B L + FC2 L − P L = 0 2 F B + 2 FC = 1.5 P ΔC = 2 ΔB FC k = 2 FB k F C = 2FB 5 F B = 1.5 P F B = 0.3 P F c = 0.6 P ΔC = 0.6 P k Problem 5-53 The rod supports a weight W and is pinned at its end A. If it is also subjected to a couple moment of M, determine the angle θ for equilibrium.The spring has an unstretched length δ and a stiffness k. 385 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 47. Engineering Mechanics - Statics Chapter 5 Given: W = 200 lb M = 100 lb ft δ = 2 ft k = 50 lb ft a = 3 ft b = 3 ft c = 2 ft Solution: Initial Guess: θ = 10 deg Given k⎡( a + b)sin ( θ ) + c − δ⎤ ( a + b) cos ( θ ) − W a cos ( θ ) − M = 0 ⎣ ⎦ θ = Find ( θ ) θ = 23.2 deg Problem 5-54 The smooth pipe rests against the wall at the points of contact A, B, and C. Determine the reactions at these points needed to support the vertical force F . Neglect the pipe's thickness in the calculation. Given: F = 45 lb 386 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 48. Engineering Mechanics - Statics Chapter 5 θ = 30 deg a = 16 in b = 20 in c = 8 in Solution: R A = 1 lb Initial Guesses: R B = 1 lb R C = 1 lb Given F cos ( θ ) ( a + b) − F sin ( θ ) c − RC b + R B c tan ( θ ) = 0 ΣMA = 0; R C cos ( θ ) − R B cos ( θ ) − F = 0 + ↑Σ Fy = 0; + → Σ Fx = 0; R A + RB sin ( θ ) − RC sin ( θ ) = 0 ⎛ RA ⎞ ⎜ ⎟ ⎜ RB ⎟ = Find ( RA , RB , RC) ⎜R ⎟ ⎝ C⎠ ⎛ RA ⎞ ⎛ 25.981 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ RB ⎟ = ⎜ 11.945 ⎟ lb ⎜ R ⎟ ⎝ 63.907 ⎠ ⎝ C⎠ Problem 5-55 The rigid metal strip of negligible weight is used as part of an electromagnetic switch. If the stiffness of the springs at A and B is k, and the strip is originally horizontal when the springs are unstretched, determine the smallest force needed to close the contact gap at C. Units Used: mN = 10 −3 N 387 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 49. Engineering Mechanics - Statics Chapter 5 Given: a = 50 mm b = 50 mm c = 10 mm k = 5 N m Solution: Initial Guesses: F = 0.5 N yA = 1 mm yB = 1 mm Given c − yA a+b = yB − yA a ⎛ yA ⎞ ⎜ ⎟ ⎜ yB ⎟ = Find ( yA , yB , F) ⎜F⎟ ⎝ ⎠ k yA + k yB − F = 0 ⎛ yA ⎞ ⎛ −2 ⎞ ⎜ ⎟ = ⎜ ⎟ mm ⎝ yB ⎠ ⎝ 4 ⎠ k yB a − F( a + b) = 0 F = 10 mN Problem 5-56 The rigid metal strip of negligible weight is used as part of an electromagnetic switch. Determine the maximum stiffness k of the springs at A and B so that the contact at C closes when the vertical force developed there is F . Originally the strip is horizontal as shown. Units Used: mN = 10 −3 N Given: a = 50 mm b = 50 mm c = 10 mm F = 0.5 N 388 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 50. Engineering Mechanics - Statics Chapter 5 Solution: Initial Guesses: k = 1 N yA = 1 mm m yB = 1 mm Given c − yA a+b = yB − yA k yA + k yB − F = 0 a ⎛ yA ⎞ ⎜ ⎟ ⎜ yB ⎟ = Find ( yA , yB , k) ⎜k ⎟ ⎝ ⎠ ⎛ yA ⎞ ⎛ −2 ⎞ ⎜ ⎟ = ⎜ ⎟ mm ⎝ yB ⎠ ⎝ 4 ⎠ k yB a − F( a + b) = 0 k = 250 N m Problem 5-57 Determine the distance d for placement of the load P for equilibrium of the smooth bar in the position θ as shown. Neglect the weight of the bar. Solution: + ↑Σ Fy = 0; Σ MA = 0; R cos ( θ ) − P = 0 −P d cos ( θ ) + R R d cos ( θ ) = R 2 d= a cos ( θ ) =0 a cos ( θ ) a cos ( θ ) 3 Problem 5-58 The wheelbarrow and its contents have mass m and center of mass at G. Determine the greatest 389 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 51. Engineering Mechanics - Statics Chapter 5 angle of tilt θ without causing the wheelbarrow to tip over. g Solution: Require point G to be over the wheel axle for tipping. Thus b cos ( θ ) = a sin ( θ ) − 1⎛ b ⎞ θ = tan ⎜ ⎟ ⎝ a⎠ Problem 5-59 Determine the force P needed to pull the roller of mass M over the smooth step. Given: M = 50 kg a = 0.6 m b = 0.1 m θ = 60 deg θ 1 = 20 deg g = 9.81 m 2 s 390 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 52. Engineering Mechanics - Statics Chapter 5 Solution: φ = acos ⎛ ⎜ a − b⎞ ⎟ ⎝ a ⎠ φ = 33.56 deg ΣMB = 0, M g sin ( θ 1 ) ( a − b) + M g cos ( θ 1 ) a sin ( φ ) ... = 0 + P cos ( θ ) ( a − b) − P sin ( θ ) a sin ( φ ) ⎡sin ( θ 1 ) ( a − b) + cos ( θ 1 ) a sin ( φ )⎤ ⎥ ⎣ cos ( θ ) ( a − b) + sin ( θ ) a sin ( φ ) ⎦ P = M g⎢ P = 441 N Problem 5-60 Determine the magnitude and direction θ of the minimum force P needed to pull the roller of mass M over the smooth step. Given: a = 0.6 m b = 0.1 m θ 1 = 20 deg M = 50 kg g = 9.81 m 2 s Solution: For Pmin, NA tends to 0 φ = acos ⎛ ⎜ a − b⎞ ⎟ ⎝ a ⎠ φ = 33.56 deg ΣMB = 0 M g sin ( θ 1 ) ( a − b) + M g cos ( θ 1 ) a sin ( φ ) ... = 0 + ⎡−P cos ( θ ) ( a − b)⎤ − P sin ( θ ) a sin ( φ ) ⎣ ⎦ 391 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 53. Engineering Mechanics - Statics P= Chapter 5 M g⎡sin ( θ 1 ) ( a − b) + cos ( θ 1 ) a sin ( φ )⎤ ⎣ ⎦ cos ( θ ) ( a − b) + a sin ( φ ) sin ( θ ) For Pmin : dP dθ = M g⎡sin ( θ 1 ) ( a − b) + cos ( θ 1 ) a sin ( φ )⎤ ⎣ ⎦ ⎣ ⎡cos ( θ ) ( a − b) + a sin ( φ ) sin ( θ )⎤ ⎦ θ = atan ⎛ sin ( φ ) ⋅ ⎜ which gives, P = ⎝ a 2 ⎣ ⎡a sin ( φ ) cos ( θ ) − ( a − b)sin ( θ )⎤ = 0 ⎦ ⎞ ⎟ θ = 33.6 deg a − b⎠ M g⎡sin ( θ 1 ) ( a − b) + cos ( θ 1 ) a sin ( φ )⎤ ⎣ ⎦ cos ( θ ) ( a − b) + a sin ( φ ) sin ( θ ) P = 395 N Problem 5-61 A uniform glass rod having a length L is placed in the smooth hemispherical bowl having a radius r. Determine the angle of inclination θ for equilibrium. Solution: By Observation φ = θ. Equilibirium : ΣMA = 0; NB2 r cos ( θ ) − W NB = L 2 cos ( θ ) = 0 WL 4r ΣF x = 0; NA cos ( θ ) − W sin ( θ ) = 0 NA = W tan ( θ ) 392 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 54. Engineering Mechanics - Statics Chapter 5 ΣF y = 0; W tan ( θ ) sin ( θ ) + WL 4r − W cos ( θ ) = 0 sin ( θ ) − cos ( θ ) = 1 − 2 cos ( θ ) = 2 2 2 cos ( θ ) − 2 cos ( θ ) = L 4r L+ 2 −L 4r cos ( θ ) cos ( θ ) − 1 = 0 2 ⎛ L + L2 + 128 r2 ⎞ ⎟ 16 r ⎝ ⎠ 2 L + 128 r θ = acos ⎜ 16 r Problem 5-62 The disk has mass M and is supported on the smooth cylindrical surface by a spring having stiffness k and unstretched length l0. The spring remains in the horizontal position since its end A is attached to the small roller guide which has negligible weight. Determine the angle θ to the nearest degree for equilibrium of the roller. Given: M = 20 kg k = 400 N m l0 = 1 m r = 2m g = 9.81 m 2 s a = 0.2 m Guesses F = 10 N Solution: R = 10 N θ = 30 deg Given + → Σ Fy = 0; R sin ( θ ) − M g = 0 + R cos ( θ ) − F = 0 ↑Σ Fx = 0; Spring F = k⎡( r + a)cos ( θ ) − l0⎤ ⎣ ⎦ 393 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 55. Engineering Mechanics - Statics ⎛F⎞ ⎜ R ⎟ = Find ( F , R , θ ) ⎜ ⎟ ⎝θ ⎠ Chapter 5 ⎛ F ⎞ ⎛ 163.633 ⎞ ⎜ ⎟=⎜ ⎟N ⎝ R ⎠ ⎝ 255.481 ⎠ θ = 50.171 deg There is also another answer that we can find by choosing different starting guesses. Guesses F = 200 N R = 200 N θ = 20 deg Solution: + → Σ Fy = 0; + ↑Σ Fx = 0; Spring Given R sin ( θ ) − M g = 0 R cos ( θ ) − F = 0 F = k⎡( r + a)cos ( θ ) − l0⎤ ⎣ ⎦ ⎛F⎞ ⎜ R ⎟ = Find ( F , R , θ ) ⎜ ⎟ ⎝θ ⎠ ⎛ F ⎞ ⎛ 383.372 ⎞ ⎜ ⎟=⎜ ⎟N ⎝ R ⎠ ⎝ 430.66 ⎠ θ = 27.102 deg Problem 5-63 Determine the x, y, z components of reaction at the fixed wall A.The force F2 is parallel to the z axis and the force F1 is parallel to the y axis. Given: a = 2m d = 2m b = 1m F 1 = 200 N c = 2.5 m F 2 = 150 N 394 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 56. Engineering Mechanics - Statics Chapter 5 Solution: ΣF x = 0; Ax = 0 ΣF x = 0; Ay = −F1 Ay = −200 N ΣF x = 0; Az = F2 Az = 150 N ΣΜx = 0; MAx = −F2 a + F 1 d MAx = 100 N⋅ m ΣΜy = 0; MAy = 0 ΣMz = 0; MAz = F 1 c MAz = 500 N⋅ m Problem 5-64 The wing of the jet aircraft is subjected to thrust T from its engine and the resultant lift force L. If the mass of the wing is M and the mass center is at G, determine the x, y, z components of reaction where the wing is fixed to the fuselage at A. Units Used: 3 Mg = 10 kg 3 kN = 10 N g = 9.81 m 2 s 395 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 57. Engineering Mechanics - Statics Chapter 5 Given: T = 8 kN L = 45 kN M = 2.1 Mg a = 2.5 m b = 5m 395 c = 3m d = 7m Solution: ΣF x = 0; − Ax + T = 0 Ax = T Ax = 8 kN ΣF y = 0; Ay = 0 Ay = 0 ΣF z = 0; − Az − M g + L = 0 Az = L − M g ΣMy = 0; Az = 24.4 kN M y − T ( a) = 0 My = T a ΣMx = 0; My = 20.0 kN⋅ m L( b + c + d) − M g b − Mx = 0 M x = L ( b + c + d) − M g b ΣMz = 0; Mx = 572 kN⋅ m Mz − T( b + c) = 0 Mz = T( b + c) Mz = 64.0 kN⋅ m Problem 5-65 The uniform concrete slab has weight W. Determine the tension in each of the three parallel supporting cables when the slab is held in the horizontal plane as shown. 396 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 58. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kip = 10 lb Given: W = 5500 lb a = 6 ft b = 3 ft c = 3 ft d = 6 ft Solution: Equations of Equilibrium : The cable tension TB can be obtained directly by summing moments about the y axis. ΣMy = 0; ΣMx = 0; W d 2 − TB d = 0 TC a + TB( a + b) − W⎛ ⎜ ⎝ TC = ΣF z = 0; TB = W 2 TB = 2.75 kip a + b + c⎞ ⎟=0 ⎠ 2 1⎡ a + b + c ⎤ − TB( a + b)⎥ ⎢W a⎣ 2 ⎦ TA + TB + TC − W = 0 TA = − TB − TC + W TC = 1.375 kip TA = 1.375 kip 397 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 59. Engineering Mechanics - Statics Chapter 5 Problem 5-66 The air-conditioning unit is hoisted to the roof of a building using the three cables. If the tensions in the cables are TA, TB and TC, determine the weight of the unit and the location (x, y) of its center of gravity G. Given: TA = 250 lb TB = 300 lb TC = 200 lb a = 5 ft b = 4 ft c = 3 ft d = 7 ft e = 6 ft Solution: ΣF z = 0; TA + TB + TC − W = 0 W = TA + TB + TC ΣMy = 0; W x − T A ( c + d) − T C d = 0 x = ΣMx = 0; W = 750 lb TA( c + d) + TC d W x = 5.2 ft TA a + TB( a + b − e) + TC( a + b) − W y = 0 y = TA a + TB( a + b − e) + TC( a + b) W y = 5.267 ft Problem 5-67 The platform truck supports the three loadings shown. Determine the normal reactions on each of its three wheels. 398 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 60. Engineering Mechanics - Statics Chapter 5 Given: F 1 = 380 lb F 2 = 500 lb F 3 = 800 lb a = 8 in b = 12 in c = 10 in d = 5 in e = 12 in f = 12 in Solution: The initail guesses are F A = 1 lb F B = 1 lb F C = 1 lb Given ΣMx = 0; F 1 ( c + d) + F2 ( b + c + d) + F3 d − F A( a + b + c + d) = 0 ΣMy = 0; F1 e − FB e − F2 f + FC f = 0 ΣF y = 0; FB + FC − F2 + FA − F1 − F3 = 0 ⎛ FA ⎞ ⎜ ⎟ ⎜ FB ⎟ = Find ( FA , FB , FC) ⎜F ⎟ ⎝ C⎠ ⎛ FA ⎞ ⎛ 663 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ FB ⎟ = ⎜ 449 ⎟ lb ⎜ F ⎟ ⎝ 569 ⎠ ⎝ C⎠ Problem 5-68 Due to an unequal distribution of fuel in the wing tanks, the centers of gravity for the airplane fuselage A and wings B and C are located as shown. If these components have weights WA, WB and WC, determine the normal reactions of the wheels D, E, and F on the ground. 399 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 61. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kip = 10 lb Given: WA = 45000 lb WB = 8000 lb WC = 6000 lb a = 8 ft e = 20 ft b = 6 ft f = 4 ft c = 8 ft g = 3 ft d = 6 ft Solution: Initial guesses: R D = 1 kip R E = 1 kip R F = 1 kip Given ΣMx = 0; WB b − RD( a + b) − WC c + R E( c + d) = 0 ΣMy = 0; W B f + W A( g + f ) + W C f − R F( e + g + f ) = 0 ΣF z = 0; R D + R E + RF − WA − WB − WC = 0 ⎛ RD ⎞ ⎜ ⎟ ⎜ RE ⎟ = Find ( RD , RE , RF) ⎜R ⎟ ⎝ F⎠ ⎛ RD ⎞ ⎛ 22.6 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ RE ⎟ = ⎜ 22.6 ⎟ kip ⎜ R ⎟ ⎝ 13.7 ⎠ ⎝ F⎠ Problem 5-69 If the cable can be subjected to a maximum tension T, determine the maximum force F which may be applied to the plate. Compute the x, y, z components of reaction at the hinge A for this loading. 400 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 62. Engineering Mechanics - Statics Chapter 5 Given: a = 3 ft b = 2 ft c = 1 ft d = 3 ft e = 9 ft T = 300 lb Solution: Initial guesses: F = 10 lb MAx = 10 lb ft MAz = 10 lb ft Ax = 10 lb Ay = 10 lb Az = 10 lb Given ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ + ⎜ 0 ⎟ = 0 ⎜ A ⎟ ⎝T − F⎠ ⎝ z⎠ ⎛ MAx ⎞ ⎛ a ⎞ ⎛ 0 ⎞ ⎛ e ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ 0 ⎟ + ⎜ −c ⎟ × ⎜ 0 ⎟ + ⎜ −b − c ⎟ × ⎜ 0 ⎟ = 0 ⎜ MAz ⎟ ⎝ 0 ⎠ ⎝ −F ⎠ ⎝ 0 ⎠ ⎝ T ⎠ ⎝ ⎠ ⎛ F ⎞ ⎜ A ⎟ ⎜ x ⎟ ⎜ Ay ⎟ ⎜ ⎟ = Find ( F , Ax , Ay , Az , MAx , MAz) Az ⎜ ⎟ ⎜ MAx ⎟ ⎟ ⎜ ⎝ MAz ⎠ ⎛ MAx ⎞ ⎛ 0 ⎞ ⎜ ⎟ = ⎜ ⎟ lb ft ⎝ MAz ⎠ ⎝ 0 ⎠ ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 0 ⎟ lb ⎜ A ⎟ ⎝ 600 ⎠ ⎝ z⎠ F = 900 lb Problem 5-70 The boom AB is held in equilibrium by a ball-and-socket joint A and a pulley and cord system as shown. Determine the x, y, z components of reaction at A and the tension in cable DEC. 401 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 63. Engineering Mechanics - Statics Chapter 5 Given: F ⎛ 0 ⎞ = ⎜ 0 ⎟ lb ⎜ ⎟ ⎝ −1500 ⎠ a = 5 ft b = 4 ft c = b d = 5 ft e = 5 ft f = 2 ft Solution: α = atan ⎛ ⎜ a ⎞ ⎟ ⎝ d + e⎠ L = 2 a + ( d + e) β = atan ⎛ ⎜ 2 ⎞ ⎟ fL ⎜L − ⎟ d+ e⎠ ⎝ Guesses b TBE = 1 lb TDEC = 1 lb Ax = 1 lb Ay = 1 lb Given Az = 1 lb 2 TDEC cos ( β ) = TBE 0 ⎞ ⎛0⎞ ⎛ 0 ⎞ ⎛ ⎜ ⎜ d ⎟ × F + ⎜ d + e ⎟ × −TBE cos ( α ) ⎟ = 0 ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎜ T sin ( α ) ⎟ ⎝0⎠ ⎝ 0 ⎠ ⎝ BE ⎠ ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ + F + TBE⎜ −cos ( α ) ⎟ = 0 ⎜A ⎟ ⎝ sin ( α ) ⎠ ⎝ z⎠ 402 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 64. Engineering Mechanics - Statics Chapter 5 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ ⎜ Az ⎟ = Find ( A , A , A , T , T x y z BE DEC) TDEC = 919 lb ⎜ ⎟ ⎜ TBE ⎟ ⎜T ⎟ ⎝ DEC ⎠ ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 1.5 × 103 ⎟ lb ⎜A ⎟ ⎜ ⎟ ⎝ z ⎠ ⎝ 750 ⎠ Problem 5-71 The cable CED can sustain a maximum tension Tmax before it fails. Determine the greatest vertical force F that can be applied to the boom. Also, what are the x, y, z components of reaction at the ball-and-socket joint A? Given: Tmax = 800 lb a = 5 ft b = 4 ft c = b d = 5 ft e = 5 ft f = 2 ft Solution: a ⎞ ⎟ ⎝ d + e⎠ α = atan ⎛ ⎜ L = 2 a + ( d + e) β = atan ⎛ ⎜ 2 ⎞ fL ⎟ ⎜L − ⎟ d+ e⎠ ⎝ Guesses b TBE = 1 lb Ax = 1 lb Ay = 1 lb Given TDEC = Tmax F = 1 lb Az = 1 lb 2 TDEC cos ( β ) = TBE 403 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 65. Engineering Mechanics - Statics Chapter 5 0 ⎞ ⎛0⎞ ⎛ 0 ⎞ ⎛ 0 ⎞ ⎛ ⎜ ⎜ d ⎟ × ⎜ 0 ⎟ + ⎜ d + e ⎟ × −TBE cos ( α ) ⎟ = 0 ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎜ T sin ( α ) ⎟ ⎝ 0 ⎠ ⎝ −F ⎠ ⎝ 0 ⎠ ⎝ BE ⎠ ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ ⎜ A ⎟ = Find ( A , A , A , T , F) x y z BE ⎜ z ⎟ ⎜ TBE ⎟ ⎜ ⎟ ⎝ F ⎠ ⎛ Ax ⎞ ⎛ 0 ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ + ⎜ 0 ⎟ + TBE⎜ −cos ( α ) ⎟ = 0 ⎜ A ⎟ ⎝ −F ⎠ ⎝ sin ( α ) ⎠ ⎝ z⎠ F = 1306 lb 0 ⎛ Ax ⎞ ⎛ ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 1.306 × 103 ⎟ lb ⎜A ⎟ ⎜ ⎟ ⎝ z ⎠ ⎝ 653.197 ⎠ Problem 5-72 The uniform table has a weight W and is supported by the framework shown. Determine the smallest vertical force P that can be applied to its surface that will cause it to tip over. Where should this force be applied? Given: W = 20 lb a = 3.5 ft b = 2.5 ft c = 3 ft e = 1.5 ft f = 1 ft Solution: f⎞ θ = atan ⎛ ⎟ ⎜ θ = 33.69 deg d = e sin ( θ ) d = 0.832 ft ⎛ a −e⎞ ⎜ 2 ⎟ φ = atan ⎜ ⎟ ⎜ b ⎟ ⎝ 2 ⎠ φ = 11.31 deg ⎝ e⎠ 404 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 66. Engineering Mechanics - Statics 2 d' = ⎛ a − e⎞ + ⎛ b ⎞ ⎜ ⎟ ⎜ ⎟ ⎝ 2 ⎠ ⎝ 2⎠ Chapter 5 2 d' = 1.275 ft Tipping will occur about the g - g axis. Require P to be applied at the corner of the table for Pmin. W d = P d' sin ( 90 deg − φ + θ ) P = W d P = 14.1 lb d' sin ( 90 deg − φ + θ ) Problem 5-73 The windlass is subjected to load W. Determine the horizontal force P needed to hold the handle in the position shown, and the components of reaction at the ball-and-socket joint A and the smooth journal bearing B. The bearing at B is in proper alignment and exerts only force reactions perpendicular to the shaft on the windlass. Given: W = 150 lb a = 2 ft b = 2 ft c = 1 ft d = 1 ft e = 1 ft f = 0.5 ft Solution: ΣMy = 0; W f−P d=0 P = W f d ΣF y = 0; Ay = 0 lb ΣMx = 0; P = 75 lb Ay = 0 −W a + B z( a + b) = 0 405 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 67. Engineering Mechanics - Statics Bz = ΣF z = 0; Chapter 5 Wa B z = 75 lb a+b Az + Bz − W = 0 Az = W − Bz ΣMz = 0; B x( a + b) − ( a + b + c + e)P = 0 Bx = ΣF x = 0; Az = 75 lb P ( a + b + c + e) a+b B x = 112 lb Ax − Bx + P = 0 Ax = Bx − P Ax = 37.5 lb Problem 5-74 A ball of mass M rests between the grooves A and B of the incline and against a vertical wall at C. If all three surfaces of contact are smooth, determine the reactions of the surfaces on the ball. Hint: Use the x, y, z axes, with origin at the center of the ball, and the z axis inclined as shown. Given: M = 2 kg θ 1 = 10 deg θ 2 = 45 deg Solution: ΣF x = 0; F c cos ( θ 1 ) − M g sin ( θ 1 ) = 0 F c = M g⋅ tan ( θ 1 ) F c = 0.32 kg⋅ m 406 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 68. Engineering Mechanics - Statics Chapter 5 ΣF y = 0; NA cos ( θ 2 ) − NB cos ( θ 2 ) = 0 NA = NB ΣF z = 0; 2 NA sin ( θ 2 ) − M g cos ( θ 1 ) − F c sin ( θ 1 ) = 0 NA = 1 M g⋅ cos ( θ 1 ) + F c⋅ sin ( θ 1 ) ⋅ 2 sin ( θ 2 ) NA = 1.3 kg⋅ m N = NA = NB Problem 5-75 Member AB is supported by cable BC and at A by a square rod which fits loosely through the square hole at the end joint of the member as shown. Determine the components of reaction at A and the tension in the cable needed to hold the cylinder of weight W in equilibrium. Units Used: 3 kip = 10 lb Given: W = 800 lb a = 2 ft b = 6 ft c = 3 ft Solution: ⎛ ⎞=0 ⎝ c +b +a ⎠ ΣF x = 0 F BC ⎜ ΣF y = 0 Ay = 0 c 2 2 2⎟ Ay = 0lb F BC = 0 lb Ay = 0 lb 407 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 69. Engineering Mechanics - Statics Chapter 5 ΣF z = 0 Az − W = 0 ΣMx = 0 MAx − W b = 0 Az = W Az = 800 lb MAx = W b MAy = 0 lb ft ΣMz = 0 MAy = 0 lb⋅ ft MAz = 0 lb ft ΣMy = 0 MAx = 4.80 kip⋅ ft MAz = 0 lb⋅ ft Problem 5-76 The pipe assembly supports the vertical loads shown. Determine the components of reaction at the ball-and-socket joint A and the tension in the supporting cables BC and BD. Units Used: 3 kN = 10 N Given: F 1 = 3 kN d = 2 m F 2 = 4 kN e = 1.5 m a = 1m g = 1m b = 1.5 m h = 3m c = 3m i = 2m f = c−e j = 2m Solution: The initial guesses are: TBD = 1 kN TBC = 1 kN Ax = 1 kN Ay = 1 kN Az = 1 kN The vectors 408 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 70. Engineering Mechanics - Statics ⎛ 0 r1 = ⎜ a + b + ⎜ ⎝ d rBC ⎞ f⎟ ⎟ ⎠ ⎛ i ⎞ = ⎜ −a ⎟ ⎜ ⎟ ⎝h − g⎠ uBC = Given rBC rBC Chapter 5 ⎛ 0 ⎞ r2 = ⎜ a + b + c ⎟ ⎜ ⎟ ⎝ d ⎠ rBD ⎛ −j ⎞ = ⎜ −a ⎟ ⎜ ⎟ ⎝h − g⎠ uBD = rBD rBD rAB ⎛0⎞ = ⎜a⎟ ⎜ ⎟ ⎝g⎠ ⎛1⎞ i = ⎜0⎟ ⎜ ⎟ ⎝0⎠ ⎛0⎞ j = ⎜1⎟ ⎜ ⎟ ⎝0⎠ ⎛0⎞ k = ⎜0⎟ ⎜ ⎟ ⎝1⎠ Ax i + Ay j + Az k − F1 k − F2 k + TBDuBD + TBC uBC = 0 rAB × ( TBDuBD + TBCuBC) + r1 × ( −F 1 k) + r2 × ( −F 2 k) = 0 ⎛ TBD ⎞ ⎜ ⎟ ⎜ TBC ⎟ ⎜ Ax ⎟ = Find ( T , T , A , A , A ) BD BC x y z ⎜ ⎟ ⎜ Ay ⎟ ⎜ A ⎟ ⎝ z ⎠ ⎛ TBD ⎞ ⎛ 17 ⎞ ⎜ ⎟ = ⎜ ⎟ kN ⎝ TBC ⎠ ⎝ 17 ⎠ ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 11.333 ⎟ kN ⎜ A ⎟ ⎝ −15.667 ⎠ ⎝ z⎠ Problem 5-77 The hatch door has a weight W and center of gravity at G. If the force F applied to the handle at C has coordinate direction angles of α, β and γ, determine the magnitude of F needed to hold the door slightly open as shown. The hinges are in proper alignment and exert only force reactions on the door. Determine the components of these reactions if A exerts only x and z components of force and B exerts x, y, z force components. Given: W = 80 lb α = 60 deg 409 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 71. Engineering Mechanics - Statics Chapter 5 β = 45 deg γ = 60 deg a = 3 ft b = 2 ft c = 4 ft d = 3 ft Solution: Initial Guesses: Ax = 1 lb Az = 1 lb F = 1 lb B x = 1 lb B y = 1 lb B z = 1 lb Given ⎛ Ax ⎞ ⎛ Bx ⎞ ⎛ cos ( α ) ⎟ ⎛ 0 ⎞ ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ ⎜ ⎟ ⎜ 0 ⎟ + ⎜ By ⎟ + F⎜ cos ( β ) ⎟ + ⎜ 0 ⎟ = 0 ⎜ Az ⎟ ⎜ B ⎟ ⎜ cos ( γ ) ⎟ ⎝ −W ⎠ ⎝ ⎠ ⎝ ⎠ ⎝ z⎠ ⎞ ⎡ ⎞⎤ ⎛ a + b ⎞ ⎢ ⎛ cos ( α ) ⎟⎥ ⎛ a ⎞ ⎛ 0 ⎞ ⎛ 0 ⎞ ⎛ Bx ⎟ ⎜ 0 ⎟ × F⎜ cos ( β ) + ⎜ c ⎟ × ⎜ 0 ⎟ + ⎜ c + d ⎟ × ⎜ B = 0 ⎟⎥ ⎜ ⎟ ⎜ ⎟ ⎜ ⎜ ⎟ ⎢ ⎜ ⎟ ⎜ y⎟ ⎢ ⎜ cos ( γ ) ⎟⎥ ⎝ 0 ⎠ ⎝ −W ⎠ ⎝ 0 ⎠ ⎜ B ⎟ ⎝ 0 ⎠ ⎣ ⎝ ⎠⎦ ⎝ z⎠ ⎛ Ax ⎞ ⎜ ⎟ ⎜ Az ⎟ ⎜ Bx ⎟ ⎜ ⎟ = Find ( Ax , Az , Bx , By , Bz , F) ⎜ By ⎟ ⎜ Bz ⎟ ⎜ ⎟ ⎝F ⎠ ⎛ Ax ⎞ ⎛ −96.5 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ Az ⎠ ⎝ −13.7 ⎠ ⎛ Bx ⎞ ⎛ 48.5 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ By ⎟ = ⎜ −67.9 ⎟ lb ⎜ B ⎟ ⎝ 45.7 ⎠ ⎝ z⎠ F = 96 lb 410 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 72. Engineering Mechanics - Statics Chapter 5 Problem 5-78 The hatch door has a weight W and center of gravity at G. If the force F applied to the handle at C has coordinate direction angles α, β, γ determine the magnitude of F needed to hold the door slightly open as shown. If the hinge at A becomes loose from its attachment and is ineffective, what are the x, y, z components of reaction at hinge B? Given: W = 80 lb α = 60 deg β = 45 deg γ = 60 deg a = 3 ft b = 2 ft c = 4 ft d = 3 ft Solution: a ΣMy = 0; F = W ΣF x = 0; B x + F cos ( α ) = 0 cos ( γ ) ( a + b) B x = −F cos ( α ) ΣF y = 0; B y = −67.9 lb B z − W + F cos ( γ ) = 0 B z = W − F cos ( γ ) ΣMx = 0; B x = −48 lb B y + F cos ( β ) = 0 B y = −F cos ( β ) ΣF z = 0; F = 96 lb B z = 32 lb MBx + W d − F cos ( γ ) ( c + d) = 0 MBx = −W d + F cos ( γ ) ( c + d) ΣMz = 0; MBx = 96 lb⋅ ft MBz + F cos ( α ) ( c + d) + F cos ( β ) ( a + b) = 0 MBz = −F cos ( α ) ( c + d) − F cos ( β ) ( a + b) MBz = −675 lb⋅ ft 411 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 73. Engineering Mechanics - Statics Chapter 5 Problem 5-79 The bent rod is supported at A, B, and C by smooth journal bearings. Compute the x, y, z components of reaction at the bearings if the rod is subjected to forces F 1 and F 2. F1 lies in the y-z plane. The bearings are in proper alignment and exert only force reactions on the rod. Given: F 1 = 300 lb d = 3 ft F 2 = 250 lb e = 5 ft a = 1 ft α = 30 deg b = 4 ft β = 45 deg c = 2 ft θ = 45 deg Solution: The initial guesses: Ax = 100 lb Ay = 200 lb B x = 300 lb B z = 400 lb Cy = 500 lb Cz = 600 lb Given Ax + B x + F2 cos ( β ) sin ( α ) = 0 Ay + Cy − F1 cos ( θ ) + F2 cos ( β ) cos ( α ) = 0 B z + Cz − F 1 sin ( θ ) − F2 sin ( β ) = 0 F 1 cos ( θ ) ( a + b) + F 1 sin ( θ ) ( c + d) − B z d − A y b = 0 Ax b + Cz e = 0 Ax( c + d) + Bx d − Cy e = 0 412 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 74. Engineering Mechanics - Statics Chapter 5 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ ⎜ ⎟ ⎜ Bx ⎟ = Find ( A , A , B , B , C , C ) x y x z y z ⎜ Bz ⎟ ⎜ ⎟ ⎜ Cy ⎟ ⎜ Cz ⎟ ⎝ ⎠ ⎛ Ax ⎞ ⎛ 632.883 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ Ay ⎠ ⎝ −141.081 ⎠ ⎛ Bx ⎞ ⎛ −721.271 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ Bz ⎠ ⎝ 895.215 ⎠ ⎛ Cy ⎞ ⎛ 200.12 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ Cz ⎠ ⎝ −506.306 ⎠ Problem 5-80 The bent rod is supported at A, B, and C by smooth journal bearings. Determine the magnitude of F2 which will cause the reaction Cy at the bearing C to be equal to zero. The bearings are in proper alignment and exert only force reactions on the rod. Given: F 1 = 300 lb d = 3 ft Cy = 0 lb e = 5 ft a = 1 ft α = 30 deg b = 4 ft β = 45 deg c = 2 ft θ = 45 deg Solution: The initial guesses: Ax = 100 lb Ay = 200 lb B x = 300 lb B z = 400 lb F 2 = 500 lb Cz = 600 lb Given Ax + B x + F2 cos ( β ) sin ( α ) = 0 413 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 75. Engineering Mechanics - Statics Chapter 5 Ay + Cy − F1 cos ( θ ) + F2 cos ( β ) cos ( α ) = 0 B z + Cz − F 1 sin ( θ ) − F2 sin ( β ) = 0 F 1 cos ( θ ) ( a + b) + F 1 sin ( θ ) ( c + d) − B z d − A y b = 0 Ax b + Cz e = 0 Ax( c + d) + Bx d − Cy e = 0 ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ ⎜ ⎟ ⎜ Bx ⎟ = Find ( A , A , B , B , C , F ) x y x z z 2 ⎜ Bz ⎟ ⎜ ⎟ ⎜ Cz ⎟ ⎜ F2 ⎟ ⎝ ⎠ F 2 = 673.704 lb Problem 5-81 Determine the tension in cables BD and CD and the x, y, z components of reaction at the ball-and-socket joint at A. Given: F = 300 N a = 3m b = 1m c = 0.5 m d = 1.5 m Solution: rBD ⎛ −b ⎞ ⎜ ⎟ = d ⎜ ⎟ ⎝a⎠ 414 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 76. Engineering Mechanics - Statics rCD Chapter 5 ⎛ −b ⎞ = ⎜ −d ⎟ ⎜ ⎟ ⎝a⎠ Initial Guesses: TBD = 1 N TCD = 1 N Ax = 1 N Ay = 1 N Az = 1 N Given ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ rBD rCD + TCD +⎜ 0 ⎟ =0 ⎜ Ay ⎟ + TBD ⎜ ⎟ rBD rCD ⎜A ⎟ ⎝ −F ⎠ ⎝ z⎠ ⎛d⎞ ⎛d⎞ ⎛d − c⎞ ⎛ 0 ⎞ rBD ⎞ ⎜ ⎟ ⎛ rCD ⎞ ⎜ ⎜ −d ⎟ × ⎛ T ⎟ ⎜ ⎟ ⎜ BD ⎟ + ⎜ d ⎟ × ⎜TCD ⎟+⎜ 0 ⎟×⎜ 0 ⎟ = 0 ⎜ ⎟ ⎝ rBD ⎠ rCD ⎠ ⎝ ⎝0⎠ ⎝0⎠ ⎝ 0 ⎠ ⎝ −F ⎠ ⎛ TBD ⎞ ⎜ ⎟ ⎜ TCD ⎟ ⎜ Ax ⎟ = Find ( T , T , A , A , A ) BD CD x y z ⎜ ⎟ ⎜ Ay ⎟ ⎜ A ⎟ ⎝ z ⎠ ⎛ TBD ⎞ ⎛ 116.7 ⎞ ⎜ ⎟=⎜ ⎟N ⎝ TCD ⎠ ⎝ 116.7 ⎠ ⎛ Ax ⎞ ⎛ 66.7 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 0 ⎟ N ⎜ A ⎟ ⎝ 100 ⎠ ⎝ z⎠ Problem 5-82 Determine the tensions in the cables and the components of reaction acting on the smooth collar at A necessary to hold the sign of weight W in equilibrium. The center of gravity for the sign is at G. Given: W = 50 lb f = 2.5 ft a = 4 ft g = 1 ft b = 3 ft h = 1 ft 415 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 77. Engineering Mechanics - Statics Chapter 5 c = 2 ft i = 2 ft d = 2 ft j = 2 ft e = 2.5 ft k = 3 ft Solution: The initial guesses are: TBC = 10 lb Ax = 10 lb MAx = 10 lb⋅ ft TDE = 10 lb Ay = 10 lb MAy = 10 lb⋅ ft Given TDE ( a − k) 2 2 ( a − k) + i + j −i TDE 2 ( a − k) + i + j j TBC 2 2 2 2 TBC 2 2 2 + Ax = 0 + Ay = 0 −W=0 2 2 ( d − b) + i + c i 2 2 ( a − k) + i + j MAy − TDE k 2 ( d − b) + i + c +c ( a − k) + i + j MAx + TDE j 2 ( d − b) + i + c 2 TDE 2 2 −i 2 TBC + ( −b + d) 2 j 2 2 ( a − k) + i + j 2 i + c TBC 2 − Wi = 0 2 2 2 2 ( d − b) + i + c d + TBC c 2 + W ( k − f) = 0 ( d − b) + i + c 416 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 78. Engineering Mechanics - Statics Chapter 5 i −TDE a 2 2 ( a − k) + i + j 2 + TBC b i 2 =0 2 2 ( d − b) + i + c ⎛ MAx ⎞ ⎜ ⎟ ⎜ MAy ⎟ ⎜ ⎟ ⎜ TBC ⎟ = Find ( M , M , T , T , A , A ) Ax Ay BC DE x y ⎜ TDE ⎟ ⎜ ⎟ ⎜ Ax ⎟ ⎜ Ay ⎟ ⎝ ⎠ ⎛ TBC ⎞ ⎛ 42.857 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ TDE ⎠ ⎝ 32.143 ⎠ ⎛ Ax ⎞ ⎛ 3.571 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ Ay ⎠ ⎝ 50 ⎠ ⎛ MAx ⎞ ⎛ 2.698 × 10− 13 ⎞ ⎜ ⎟=⎜ ⎟ lb⋅ ft ⎝ MAy ⎠ ⎝ −17.857 ⎠ Problem 5-83 The member is supported by a pin at A and a cable BC. If the load at D is W, determine the x, y, z components of reaction at these supports. Units Used: 3 kip = 10 lb Given: W = 300 lb a = 1 ft b = 2 ft c = 6 ft d = 2 ft e = 2 ft f = 2 ft 417 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 79. Engineering Mechanics - Statics Chapter 5 Solution: Initial Guesses: TBC = 1 lb Ax = 1 lb Ay = 1 lb Az = 1 lb MAy = 1 lb ft MAz = 1 lb ft Given ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ + ⎜A ⎟ ⎝ z⎠ ⎛e + f − a⎞ ⎛ 0 ⎞ ⎜ −c ⎟ + ⎜ 0 ⎟ = 0 ⎟ ⎜ ⎟ 2 2 2⎜ b + c + ( e + f − a) ⎝ b ⎠ ⎝ −W ⎠ TBC ⎛ 0 ⎞ ⎛ −e ⎞ ⎛ 0 ⎞ ⎛ −a ⎞ ⎡ ⎛ e + f − a ⎞⎤ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎢ TBC ⎜ −c ⎟⎥ = 0 ⎜ MAy ⎟ + ⎜ c ⎟ × ⎜ 0 ⎟ + ⎜ 0 ⎟ × ⎢ ⎜ ⎟⎥ ⎜ MAz ⎟ ⎝ 0 ⎠ ⎝ −W ⎠ ⎝ b ⎠ ⎣ b2 + c2 + ( e + f − a) 2 ⎝ b ⎠⎦ ⎝ ⎠ ⎛ TBC ⎞ ⎜ ⎟ ⎜ Ax ⎟ ⎜ ⎟ ⎜ Ay ⎟ = Find ( T , A , A , A , M , M ) BC x y z Ay Az ⎜ Az ⎟ ⎜ ⎟ ⎜ MAy ⎟ ⎜ MAz ⎟ ⎝ ⎠ TBC = 1.05 kip ⎛ Ax ⎞ ⎛ −450 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 900 ⎟ lb ⎜A ⎟ ⎝ 0 ⎠ ⎝ z⎠ ⎛ MAy ⎞ ⎛ −600 ⎞ ⎜ ⎟=⎜ ⎟ lb⋅ ft ⎝ MAz ⎠ ⎝ −900 ⎠ Problem 5-84 Determine the x, y, z components of reaction at the pin A and the tension in the cable BC necessary for equilibrium of the rod. 418 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 80. Engineering Mechanics - Statics Chapter 5 Given: F = 350 lb e = 12 ft a = 4 ft f = 4 ft b = 5 ft g = 10 ft c = 4 ft h = 4 ft d = 2 ft i = 10 ft Solution: Initial Guesses: F BC = 1 lb Ay = 1 lb MAy = 1 lb⋅ ft Ax = 1 lb Az = 1 lb MAz = 1 lb⋅ ft Given ⎛ 0 ⎞ ⎛d⎞ ⎡ ⎛ g − d ⎞⎤ ⎜ ⎟ ⎜ ⎟ ⎢ F ⎜ e − c ⎟⎥ ... = 0 ⎜ MAy ⎟ + ⎜ c ⎟ × ⎢ ⎟⎥ 2 2 2⎜ ⎜ MAz ⎟ ⎝ 0 ⎠ ⎣ ( g − d) + ( e − c) + f ⎝ − f ⎠⎦ ⎝ ⎠ ⎛ −a ⎞ ⎡ ⎛ a − h ⎞⎤ F BC ⎜ 0 ⎟×⎢ ⎜ −e ⎟⎥ + ⎜ ⎟ ⎢ ⎟⎥ 2 2 2⎜ ⎝ b ⎠ ⎣ ( a − h) + e + b ⎝ b ⎠⎦ ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ + ⎜A ⎟ ⎝ z⎠ ⎛g − d⎞ ⎜e− c⎟ + ⎟ 2 2 2⎜ ( g − d) + ( e − c) + f ⎝ − f ⎠ F ⎛a − h⎞ ⎜ −e ⎟ = 0 ⎟ 2 2 2⎜ ( a − h) + e + b ⎝ b ⎠ F BC 419 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 81. Engineering Mechanics - Statics Chapter 5 ⎛ FBC ⎞ ⎜ ⎟ ⎜ Ax ⎟ ⎜ ⎟ ⎜ Ay ⎟ = Find ( F , A , A , A , M , M ) BC x y z Ay Az ⎜ Az ⎟ ⎜ ⎟ ⎜ MAy ⎟ ⎜ MAz ⎟ ⎝ ⎠ F BC = 101 lb ⎛ Ax ⎞ ⎛ −233.3 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ −140 ⎟ lb ⎜ A ⎟ ⎝ 77.8 ⎠ ⎝ z⎠ ⎛ MAy ⎞ ⎛ −388.9 ⎞ ⎜ ⎟=⎜ ⎟ lb⋅ ft ⎝ MAz ⎠ ⎝ 93.3 ⎠ Problem 5-85 Rod AB is supported by a ball-and-socket joint at A and a cable at B. Determine the x, y, z components of reaction at these supports if the rod is subjected to a vertical force F as shown. Given: F = 50 lb a = 2 ft c = 2 ft b = 4 ft d = 2 ft Solution: TB = 10 lb Ax = 10 lb Ay = 10 lb Az = 10 lb B y = 10 lb Given ΣF x = 0; − TB + A x = 0 ΣF y = 0; Ay + By = 0 420 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 82. Engineering Mechanics - Statics ΣF z = 0; Chapter 5 −F + A z = 0 ΣMAx = 0; F ( c) − By( b) = 0 ΣMAy = 0; F ( a) − TB( b) = 0 ΣMAz = 0; B y( a) − TB( c) = 0 Solving, ⎛ TB ⎞ ⎜ ⎟ ⎜ Ax ⎟ ⎜ Ay ⎟ = Find ( T , A , A , A , B ) B x y z y ⎜ ⎟ ⎜ Az ⎟ ⎜B ⎟ ⎝ y⎠ ⎛ TB ⎞ ⎛ 25 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ax ⎟ ⎜ 25 ⎟ ⎜ Ay ⎟ = ⎜ −25 ⎟ lb ⎜ ⎟ ⎜ ⎟ ⎜ Az ⎟ ⎜ 50 ⎟ ⎜ B ⎟ ⎝ 25 ⎠ ⎝ y⎠ Problem 5-86 The member is supported by a square rod which fits loosely through a smooth square hole of the attached collar at A and by a roller at B. Determine the x, y, z components of reaction at these supports when the member is subjected to the loading shown. Given: M = 50 lb⋅ ft ⎛ 20 ⎞ ⎜ ⎟ F = −40 lb ⎜ ⎟ ⎝ −30 ⎠ a = 2 ft b = 1 ft c = 2 ft Solution: Initial Guesses Ax = 1 lb Ay = 1 lb 421 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 83. Engineering Mechanics - Statics Chapter 5 MAx = 1 lb ft MAy = 1 lb ft MAz = 1 lb ft B z = 1 lb Given ⎛ Ax ⎞ ⎛ 0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ + ⎜ 0 ⎟ + F = 0 ⎜ 0 ⎟ ⎜ Bz ⎟ ⎝ ⎠ ⎝ ⎠ ⎛ MAx ⎞ ⎛ 0 ⎞ ⎛ 0 ⎞ ⎡⎛ 0 ⎞ ⎛ 0 ⎞⎤ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎢⎜ ⎟ × F + ⎜ 0 ⎟⎥ = 0 ⎜ MAy ⎟ + ⎜ a ⎟ × ⎜ 0 ⎟ + ⎢⎜ a + b ⎟ ⎜ ⎟⎥ ⎜ M ⎟ ⎝ 0 ⎠ ⎜ Bz ⎟ ⎣⎝ −c ⎠ ⎝ −M ⎠⎦ ⎝ ⎠ ⎝ Az ⎠ ⎛ Ax ⎞ ⎜ ⎟ ⎜ Ay ⎟ ⎜ ⎟ ⎜ MAx ⎟ = Find ( A , A , M , M , M , B ) x y Ax Ay Az z ⎜ MAy ⎟ ⎜ ⎟ ⎜ MAz ⎟ ⎜ Bz ⎟ ⎝ ⎠ ⎛ Ax ⎞ ⎛ −20 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ Ay ⎠ ⎝ 40 ⎠ B z = 30 lb ⎛ MAx ⎞ ⎛ 110 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ MAy ⎟ = ⎜ 40 ⎟ lb⋅ ft ⎜ M ⎟ ⎝ 110 ⎠ ⎝ Az ⎠ Problem 5-87 The platform has mass M and center of mass located at G. If it is lifted using the three cables, determine the force in each of these cables. Units Used: 3 Mg = 10 kg 3 kN = 10 N g = 9.81 m 2 s 422 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 84. Engineering Mechanics - Statics Chapter 5 Given: M = 3 Mg a = 4m b = 3m c = 3m d = 4m e = 2m Solution: The initial guesses are: F AC = 10 N F BC = 10 N F DE = 10 N Given b ( F AC) 2 2 a +b − c ( F BC) 2 =0 2 a +c M g e − ( F AC) a d+e 2 2 − FBC a +b a 2 2 a( d + e) 2 =0 2 a +c F BC( b + c) − M g b + FDE b = 0 a +c ⎛ FAC ⎞ ⎜ ⎟ FBC ⎟ = Find ( F AC , F BC , F DE) ⎜ ⎜F ⎟ ⎝ DE ⎠ ⎛ FAC ⎞ ⎜ ⎟ FBC ⎟ = kN ⎜ ⎜F ⎟ ⎝ DE ⎠ 423 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 85. Engineering Mechanics - Statics Chapter 5 Problem 5-88 The platform has a mass of M and center of mass located at G. If it is lifted using the three cables, determine the force in each of the cables. Solve for each force by using a single moment equation of equilibrium. Units Used: Mg = 1000 kg 3 kN = 10 N g = 9.81 m 2 s Given: M = 2 Mg c = 3m a = 4m d = 4m b = 3m e = 2m Solution: rBC ⎛0⎞ = ⎜ −c ⎟ ⎜ ⎟ ⎝a⎠ rAC rAD ⎛ −e − d ⎞ = ⎜ b ⎟ ⎜ ⎟ ⎝ 0 ⎠ ⎛0⎞ = ⎜b⎟ ⎜ ⎟ ⎝a⎠ rBD ⎛ −d − e ⎞ = ⎜ −c ⎟ ⎜ ⎟ ⎝ 0 ⎠ First find FDE. ΣMy' = 0; F DE( d + e) − M g d = 0 Next find FBC. Guess ⎡⎛ e ⎞ ⎛ 0 Given ⎢⎜ 0 ⎟ × ⎜ 0 ⎢⎜ ⎟ ⎜ ⎣⎝ 0 ⎠ ⎝ −M F DE = Mgd d+e 2 F DE = 4.1 s kN F BC = 1 kN ⎞ ⎛e + d⎞ rBC ⎟ + ⎜ c ⎟ × ⎛F ⎜ BC ⎟ ⎜ ⎟ ⎝ rBC g⎠ ⎝ 0 ⎠ ⎤ ⎞⎥ ⎟⎥ rAD = 0 ⎠ ⎦ F BC = Find ( FBC ) F BC = kN Now find FAC. Guess F AC = 1 kN 424 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 86. Engineering Mechanics - Statics ⎡⎛ e ⎞ ⎛ 0 Given ⎢⎜ 0 ⎟ × ⎜ 0 ⎢⎜ ⎟ ⎜ ⎣⎝ 0 ⎠ ⎝ −M Chapter 5 ⎞ ⎛e + d⎞ rAC ⎟ + ⎜ −b ⎟ × ⎛ F ⎜ AC ⎟ ⎜ ⎟ ⎝ rAC g⎠ ⎝ 0 ⎠ ⎤ ⎞⎥ ⎟⎥ rBD = 0 ⎠ ⎦ F AC = Find ( FAC ) F AC = kN Problem 5-89 The cables exert the forces shown on the pole. Assuming the pole is supported by a ball-and-socket joint at its base, determine the components of reaction at A. The forces F 1 and F2 lie in a horizontal plane. Given: F 1 = 140 lb F 2 = 75 lb θ = 30 deg a = 5 ft b = 10 ft c = 15 ft Solution: The initial guesses are TBC = 100 lb TBD = 100 lb Ax = 100 lb Ay = 100 lb Az = 100 lb Given c ⎞ ⎞ ⎛ ⎟ − TBD a⎜ 2 2 ⎟ = 0 2 2 2 ⎝ a +b +c ⎠ ⎝ a +c ⎠ (F1 cos (θ ) + F2)c − TBC a⎛ ⎜ c ⎞=0 ⎝ a +b +c ⎠ F 1 sin ( θ ) c − b TBC ⎛ ⎜ c 2 2 2⎟ 425 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 87. Engineering Mechanics - Statics ⎛ Chapter 5 ⎞ ⎟=0 2 2 2 a +b +c ⎠ ⎝ Ax + F 1 sin ( θ ) − b⎜ TBC TBC ⎞ ⎞ + a⎛ ⎜ 2 2 2⎟ = 0 ⎟ 2 2 ⎝ a +c ⎠ ⎝ a +b +c ⎠ ⎛ Ay − F 1 cos ( θ ) − F 2 + TBD⎜ a TBC ⎛ TBD ⎞ ⎛ ⎞ − c⎜ ⎟ ⎟=0 2 2 2 2 2 ⎝ a +c ⎠ ⎝ a +b +c ⎠ Az − c⎜ ⎛ TBC ⎞ ⎜ ⎟ ⎜ TBD ⎟ ⎜ Ax ⎟ = Find ( T , T , A , A , A ) BC BD x y z ⎜ ⎟ ⎜ Ay ⎟ ⎜ A ⎟ ⎝ z ⎠ ⎛ TBC ⎞ ⎛ 131.0 ⎞ ⎜ ⎟=⎜ ⎟ lb ⎝ TBD ⎠ ⎝ 509.9 ⎠ ⎛ Ax ⎞ ⎛ −0.0 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ = ⎜ 0.0 ⎟ lb ⎜ A ⎟ ⎝ 588.7 ⎠ ⎝ z⎠ Problem 5-90 The silo has a weight W, a center of gravity at G and a radius r. Determine the vertical component of force that each of the three struts at A, B, and C exerts on the silo if it is subjected to a resultant wind loading of F which acts in the direction shown. Given: W = 3500 lb F = 250 lb θ 1 = 30 deg θ 2 = 120 deg θ 3 = 30 deg r = 5 ft b = 12 ft c = 15 ft 426 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 88. Engineering Mechanics - Statics Chapter 5 Solution: Initial Guesses: Az = 1 lb B z = 2 lb Cz = 31 lb Given ΣMy = 0; B z r cos ( θ 1 ) − Cz r cos ( θ 1 ) − F sin ( θ 3 ) c = 0 [1] ΣMx = 0; −B z r sin ( θ 1 ) − Cz r sin ( θ 1 ) + Az r − F cos ( θ 3 ) c = 0 [2] ΣF z = 0; Az + Bz + Cz = W [3] Solving Eqs.[1], [2] and [3] yields: ⎛ Az ⎞ ⎜ ⎟ ⎜ Bz ⎟ = Find ( Az , Bz , Cz) ⎜C ⎟ ⎝ z⎠ ⎛ Az ⎞ ⎛ 1600 ⎞ ⎜ ⎟ ⎜ ⎟ ⎜ Bz ⎟ = ⎜ 1167 ⎟ lb ⎜ C ⎟ ⎝ 734 ⎠ ⎝ z⎠ Problem 5-91 The shaft assembly is supported by two smooth journal bearings A and B and a short link DC. If a couple moment is applied to the shaft as shown, determine the components of force reaction at the bearings and the force in the link. The link lies in a plane parallel to the y-z plane and the bearings are properly aligned on the shaft. Units Used: 3 kN = 10 N Given: M = 250 N⋅ m a = 400 mm b = 300 mm c = 250 mm d = 120 mm θ = 30 deg 427 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 89. Engineering Mechanics - Statics Chapter 5 φ = 20 deg Solution: Initial Guesses: Ay = 1 kN Az = 1 kN B z = 1 kN B y = 1 kN F CD = 1 kN Given 0 ⎞ ⎛0 ⎞ ⎛0⎞ ⎛ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ Ay ⎟ + ⎜ By ⎟ + ⎜ −FCD cos ( φ ) ⎟ = 0 ⎜ Az ⎟ ⎜ Bz ⎟ ⎜ F sin ( φ ) ⎟ ⎝ ⎠ ⎝ ⎠ ⎝ CD ⎠ 0 ⎞ ⎛ −a − b ⎞ ⎛ 0 ⎞ ⎛ −M ⎞ ⎛ d−a ⎞ ⎛ ⎜ ⎜ c sin ( θ ) ⎟ × −FCD cos ( φ ) ⎟ + ⎜ 0 ⎟ × ⎜ By ⎟ + ⎜ 0 ⎟ = 0 ⎟ ⎜ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ ⎟ ⎜ F sin ( φ ) ⎟ ⎝ 0 ⎠ ⎜ Bz ⎟ ⎝ 0 ⎠ ⎝ c cos ( θ ) ⎠ ⎝ CD ⎝ ⎠ ⎠ ⎛ Ay ⎞ ⎜ ⎟ Az ⎟ ⎜ ⎜ By ⎟ = Find ( A , A , B , B , F ) y z y z CD ⎜ ⎟ ⎜ Bz ⎟ ⎜F ⎟ ⎝ CD ⎠ ⎛ Ay ⎞ ⎛ 573 ⎞ ⎜ ⎟=⎜ ⎟N ⎝ Az ⎠ ⎝ −208 ⎠ ⎛ By ⎞ ⎛ 382 ⎞ ⎜ ⎟=⎜ ⎟N ⎝ Bz ⎠ ⎝ −139 ⎠ F CD = 1.015 kN Problem 5-92 If neither the pin at A nor the roller at B can support a load no greater than F max, determine the maximum intensity of the distributed load w, so that failure of a support does not occur. 428 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 90. Engineering Mechanics - Statics Chapter 5 Units Used: 3 kN = 10 N Given: F max = 6 kN a = 3m b = 3m Solution: The greatest reaction is at A. Require ΣMB = 0; −F max( a + b) + w a w = F max( a + b) a⎛ ⎜ a ⎝2 + b⎞ + ⎟ ⎠ 2 ⎛ a + b⎞ + 1 w b 2 b = 0 ⎜ ⎟ 3 ⎝2 ⎠ 2 w = 2.18 b kN m 3 Problem 5-93 If the maximum intensity of the distributed load acting on the beam is w, determine the reactions at the pin A and roller B. Units Used: 3 kN = 10 N Given: F = 6 kN a = 3m b = 3m 429 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
- 91. Engineering Mechanics - Statics w = 4 Chapter 5 kN m Solution: ΣF x = 0; Ax = 0 ΣMA = 0; −w a By = a 2 1 6 − 1 2 w b⎛ a + ⎜ ⎝ ⎟ + By( a + b) = 0 3⎠ 2 w 3a + 3 a b + b Ay + By − w a − ΣF y = 0; b⎞ 2 B y = 7 kN a+b 1 2 Ay = −By + w a + wb=0 1 2 Ay = 11 kN wb Problem 5-94 Determine the normal reaction at the roller A and horizontal and vertical components at pin B for equilibrium of the member. Units Used: 3 kN = 10 N Given: F 1 = 10 kN F 2 = 6 kN a = 0.6 m b = 0.6 m c = 0.8 m d = 0.4 m θ = 60 deg 430 © 2007 R. C. Hibbeler. Published by Pearson Education, Inc., Upper Saddle River, NJ. All rights reserved. This material is protected under all copyright laws as they currently exist. No portion of this material may be reproduced, in any form or by any means, without permission in writing from the publisher.
-

ssuser8f7e07
Sep. 22, 2021 -

MoniurRahman
Apr. 6, 2021 -

NguynBnh31
Dec. 9, 2020 -

RonaldJrGarcia
Oct. 16, 2020 -

AdesuyioluwafemiEmma
Oct. 10, 2019 -

ssuser708c66
Apr. 17, 2019 -

LinhHoang225
Mar. 14, 2019 -

JanuaKim
Feb. 15, 2019 -

AllegreGuerrero
Feb. 13, 2019 -

TahaYasin10
Dec. 23, 2018 -

ArifAfridi1
Dec. 16, 2018 -

JaraBrailles
Nov. 7, 2018 -

charlengineering
Oct. 29, 2018 -

MeoChiln
Sep. 9, 2018 -

MaximNardo
Jul. 22, 2018 -

AlessandraLeal5
Jul. 2, 2018 -

TalhaRazzaq6
Apr. 29, 2018 -

HikmetEdaAkba
Apr. 18, 2018 -

DeckAlzaga
Feb. 28, 2018 -

DevaSurya4
Feb. 22, 2018
Engineering Mechanics Statics 12th Edition Solutions Chapter 5
Source: https://www.slideshare.net/laibajaved1/hibbeler-chapter5
0 Response to "Engineering Mechanics Statics 12th Edition Solutions Chapter 5"
Post a Comment